Consider the solution to the unsteady diffusion equation

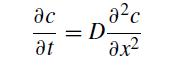
by the method of lines and centered finite differences with a spacing x. The initial condition is c(x, 0) = 0 and the left boundary condition satisfies no-flux. At the start of the process, concentration at the boundary at x = L is instantaneously increased to some value c_A. Write the ODEs and initial conditions for (i) the left node, (ii) the interior nodes, and (iii) the right node. If you solve this problem with implicit Euler, what numerical method should you use to compute the concentrations c_i^(k+1) from the values of c_i^(k)?

at = a2c D- 2