Consider the system of equations (a) Calculate the eigenvalues and eigenvectors. (b) Using matrix diagonalization, obtain (analytically)
Question:
Consider the system of equations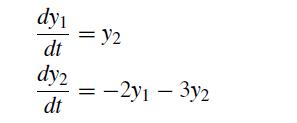
(a) Calculate the eigenvalues and eigenvectors.
(b) Using matrix diagonalization, obtain (analytically) the solution of the equations for y1(0) = y2(0) = 1.
(c) How does the solution behave for large t? Is this consistent with your eigenvalue analysis? Explain.
(d) Suppose you were using explicit Euler to integrate the system numerically. What would be the maximum integration step size to guarantee numerical stability? Explain.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answered By
Mercy Kangai
I provide creative and detailed administrative, web search, academic writing, data entry, Personal assistant, Content writing, Translation, Academic writing, editing and proofreading services. I excel at working under tight deadlines with strict expectations. I possess the self-discipline and time management skills necessary to have served as an academic writer for the past seven years. I can bring value to your business and help solve your administrative assistant issues. I have extensive experience in marketing and small business management.
27+ Reviews
86+ Question Solved
Related Book For 
Numerical Methods With Chemical Engineering Applications
ISBN: 9781107135116
1st Edition
Authors: Kevin D. Dorfman, Prodromos Daoutidis
Question Posted:
