We can get an accurate idea of the error in centered finite differences by studying the solution to an exactly solvable problem and directly calculating the error in the finite difference representation. Consider the simple reaction–diffusion problem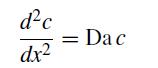

where Da is the Dämkohler number. (We have already made the problem dimensionless for you.) The system is subject to the Dirichlet boundary conditions at x = 0 and x = 1. Use the exact solution to this problem to calculate the error in the finite difference approximation at node i as a function of the exact concentration at that position, c_i = c(x = x_i), the grid spacing, Δx, and the Dämkohler number. For which values of the Dämkohler number is the finite difference approximation exact for this problem?

Transcribed Image Text:

dc dx2 = Dac