1 Farmer Leary grows wheat and corn on his 45-acre farm. He can sell at most 140 bushels of wheat and 120 bushels of corn. Each acre planted with wheat yields 5 bushels, and each acre planted with corn yields 4 bushels.

Wheat sells for $30 per bushel, and corn sells for $50 per bushel. To harvest an acre of wheat requires 6 hours of labor; 10 hours are needed to harvest an acre of corn. Up to 350 hours of labor can be purchased at $10 per hour. Let A1 = acres planted with wheat; A2 = acres planted with corn; and L= hours of labor that are purchased. To maximize profits, Leary should solve the following LP:

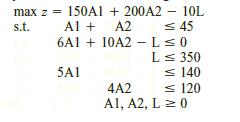
Use the LINDO output in Figure 6 to answer the following questions:
a If only 40 acres of land were available, what would Leary’s profit be?
b If the price of wheat dropped to $26, what would be the new optimal solution to Leary’s problem?
c Use the SLACK portion of the output to determine the allowable increase and allowable decrease for the amount of wheat that can be sold. If only 130 bushels of wheat could be sold, then would the answer to the problem change?

Transcribed Image Text:

max z 150A1 + 200A210L s.t. A1 + A2 6A110A2-L0 45 L 350 5A1 140 4A2 120 A1, A2, L 0