A second-degree polynomial with values p(x) = y 0 + y 1 x + y2x 2 is
Question:
A second-degree polynomial with values p(x) = y0 + y1x + y2x2 is non-negative everywhere if and only if
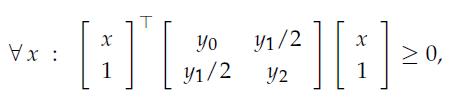
which in turn can be written as an LMI in y = (y0, y1, y2):

In this exercise, you show a more general result, which applies to any polynomial of even degree 2k (polynomials of odd degree can’t be non-negative everywhere). To simplify, we only examine the case k = 2, that is, fourth-degree polynomials; the method employed here can be generalized to k > 2.
1. Show that a fourth-degree polynomial p is non-negative everywhere if and only if it is a sum of squares, that is, it can be written as
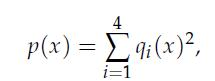
where qi’s are polynomials of degree at most two.
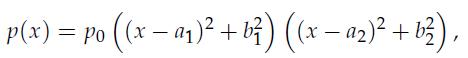
for some appropriate real numbers ai, bi, i = 1, 2, and some p0 ≥ 0.
2. Using the previous part, show that if a fourth-degree polynomial is a sum of squares, then it can be written as.

for some positive-semidefinite matrix Q.
3. Show the converse: if a positive semi-definite matrix Q satisfies condition (11.25) for every x, then p is a sum of squares.
4. Show that a fourth-degree polynomial ![]()
![]() is non-negative everywhere if and only if there exist a 5 x 5 matrix Q such that
is non-negative everywhere if and only if there exist a 5 x 5 matrix Q such that
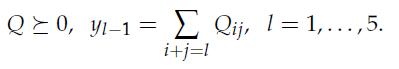
Step by Step Answer:

Optimization Models
ISBN: 9781107050877
1st Edition
Authors: Giuseppe C. Calafiore, Laurent El Ghaoui





