For X S n , and i {1, . . . , n}, we denote
Question:
For X ∈ Sn, and i ∈ {1, . . . , n}, we denote by λi(X) the i-th largest eigenvalue of X. For k ∈ {1, . . . , n}, we define the function fk : Sn → R with values
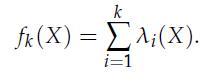
This function is an intermediate between the largest eigenvalue (obtained with k = 1) and the trace (obtained with k = n).
1. Show that for every t ∈ R, we have fk(X) ≤ t if and only if there exist Z ∈ Sn and s ∈ R such that
![]()
2. Show that fk is convex. Is it a norm?
3. How would you generalize these results to the function that assigns the sum of top k singular values to a general rectangular m x n matrix, with k ≤ min(m, n)?
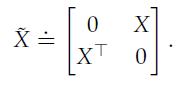
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Optimization Models
ISBN: 9781107050877
1st Edition
Authors: Giuseppe C. Calafiore, Laurent El Ghaoui
Question Posted:





