7.3.1 In another form of sum quota sampling (see Chapter 5, Section 5.4.2), a sequence of nonnegative
Question:
7.3.1 In another form of sum quota sampling (see Chapter 5, Section 5.4.2), a sequence of nonnegative independent and identically distributed random variables X1;X2; : : : is observed, the sampling continuing until the first time that the sum of the observations exceeds the quota t. In renewal process terminology, the sample size is N.t/C1. The sample mean is
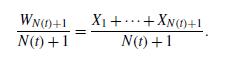
An important question in statistical theory is whether or not this sample mean is unbiased. That is, how does the expected value of this sample mean relate to the expected value of, say, X1? Assume that the individual X summands are exponentially distributed with parameter , so that N.t/ is a Poisson process, and evaluate the expected value of the foregoing sample mean and show that
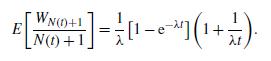
Hint: Use the result of the previous exercise, that ![]() are independent, and then evaluate separately
are independent, and then evaluate separately
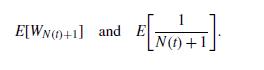
Step by Step Answer:

An Introduction To Stochastic Modeling
ISBN: 9780233814162
4th Edition
Authors: Mark A. Pinsky, Samuel Karlin






