Let (left(B_{t} ight)_{t geqslant 0}) be a (mathrm{BM}^{1}). Apply Doob's maximal inequality (A.13) to the exponential martingale
Question:
Let \(\left(B_{t}\right)_{t \geqslant 0}\) be a \(\mathrm{BM}^{1}\). Apply Doob's maximal inequality (A.13) to the exponential martingale \(M_{t}^{\xi}:=\exp \left(\xi B_{t}-\frac{1}{2} \xi^{2} t\right)\) to show that for all \(x, \xi>0\)\[\mathbb{P}\left(\sup _{s \leqslant t}\left(B_{s}-\frac{1}{2} \xi s\right)>x\right) \leqslant e^{-x \xi}\]
(This inequality can be used for the upper bound in the proof of Theorem 12.1, avoiding the combination of (12.2) and the upper bound in (12.1).)
Data From 12.1 Theorem
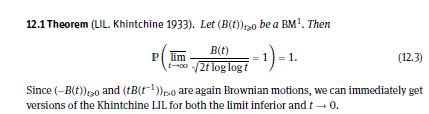
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher
Question Posted:





