Let ({X(t), t geq 0}) be the cumulative repair cost process of a system with [X(t)=0.01 e^{D(t)}]
Question:
Let \(\{X(t), t \geq 0\}\) be the cumulative repair cost process of a system with
\[X(t)=0.01 e^{D(t)}\]
where \(\{D(t), t \geq 0\}\) is a Brownian motion with drift and parameters
\[\mu=0.02 \text { and } \sigma^{2}=0.04\]
The cost of a system replacement by an equivalent new one is \(c=4000\).
(1) The system is replaced according to policy 1 (page 522). Determine the optimal repair cost limit \(x^{*}\) and the corresponding maintenance cost rate \(K_{1}\left(x^{*}\right)\).
(2) The system is replaced according to policy 2 (page 522). Determine its economic lifetime \(\tau^{*}\) based on the average repair cost development
\[E(X(t))=0.01 E\left(e^{D(t)}\right)\]
and the corresponding maintenance cost rate \(K_{2}\left(\tau^{*}\right)\).
(3) Analogously to example 11.8, apply replacement policy 2 to the cumulative repair cost process \[X(t)=0.01 e^{M(t)}\]
with \(M(t)=\max _{0 \leq y \leq t} D(y)\). Determine the corresponding economic lifetime of the system and the maintenance cost rate \(K_{2}\left(\tau^{*} \mid M\right)\). Compare to the minimal maintenance cost rates determined under (1) and (2).
For part (3) of this exercise you need computer assistance.
Data from Example 11.8
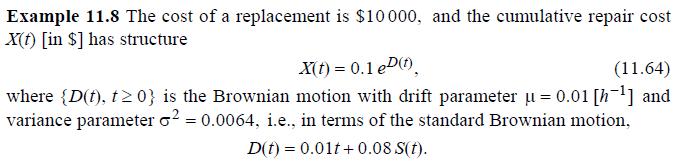
Step by Step Answer:

Applied Probability And Stochastic Processes
ISBN: 9780367658496
2nd Edition
Authors: Frank Beichelt





