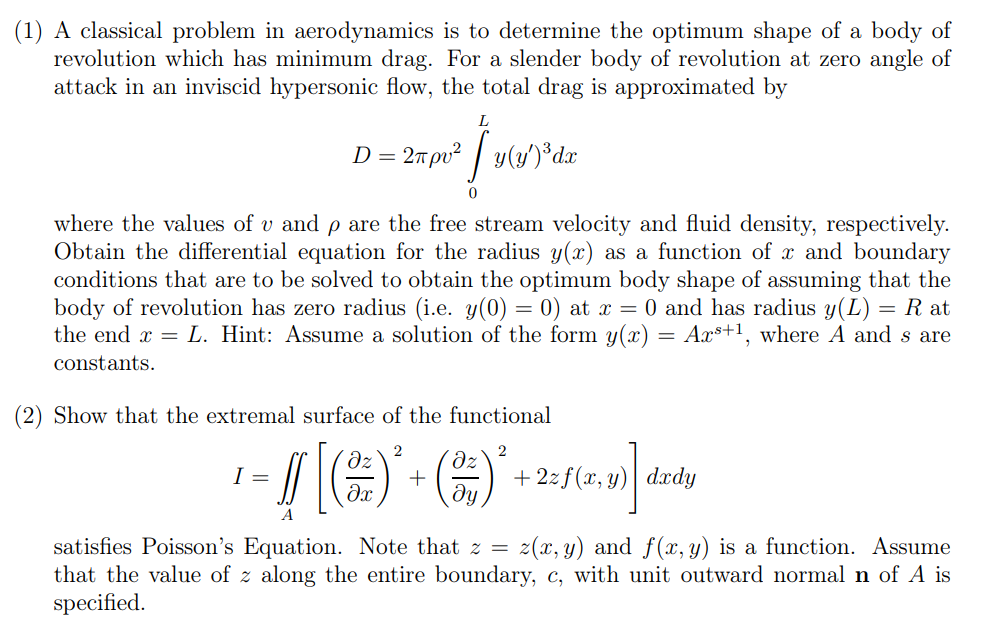
(1) A classical problem in aerodynamics is to determine the optimum shape of a body of revolution which has minimum drag. For a slender body of revolution at zero angle of attack in an inviscid hypersonic flow, the total drag is approximated by L D = 2po y(y) dr where the values of v and p are the free stream velocity and fluid density, respectively. Obtain the differential equation for the radius y(x) as a function of x and boundary conditions that are to be solved to obtain the optimum body shape of assuming that the body of revolution has zero radius (i.e. y(0) = 0) at x = 0 and has radius y(L) = R at the end x = L. Hint: Assume a solution of the form y(x) = Axs+1, where A and s are constants. (2) Show that the extremal surface of the functional I = A ()()+2= +2zf(x, y) dxdy satisfies Poisson's Equation. Note that z = = 2(x, y) and f(x, y) is a function. Assume that the value of z along the entire boundary, c, with unit outward normal n of A is specified.