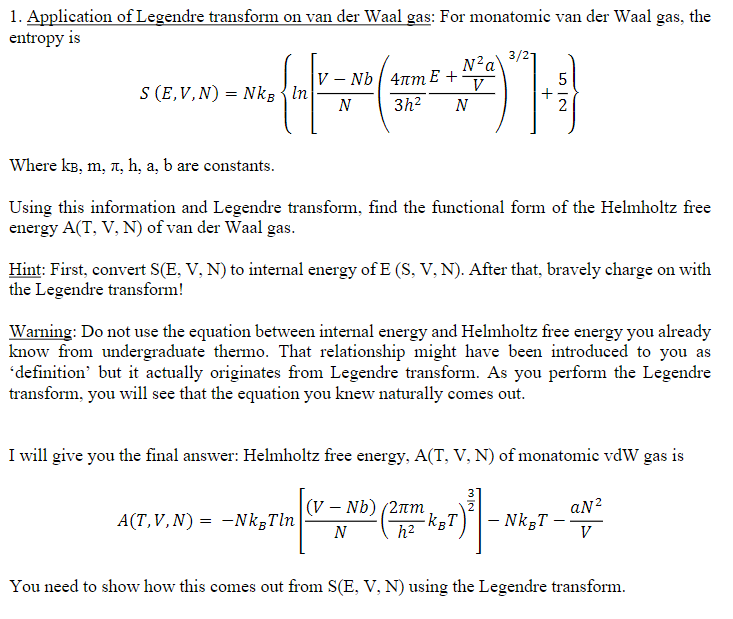
1. Application of Legendre transform on van der Waal gas: For monatomic van der Waal gas, the entropy is 3/21 N2a\ S (E,V,N) = Ng In V-Nb 4m E + N 3h2 N 5 V 2 Where KB, m, , h, a, b are constants. Using this information and Legendre transform, find the functional form of the Helmholtz free energy A(T, V, N) of van der Waal gas. Hint: First, convert S(E, V, N) to internal energy of E (S, V, N). After that, bravely charge on with the Legendre transform! Warning: Do not use the equation between internal energy and Helmholtz free energy you already know from undergraduate thermo. That relationship might have been introduced to you as 'definition' but it actually originates from Legendre transform. As you perform the Legendre transform, you will see that the equation you knew naturally comes out. I will give you the final answer: Helmholtz free energy, A(T, V, N) of monatomic vdW gas is = A(T,V,N) -NkTln [(V Nb) (2m N h .kBT - NkBT- - aN V You need to show how this comes out from S(E, V, N) using the Legendre transform.