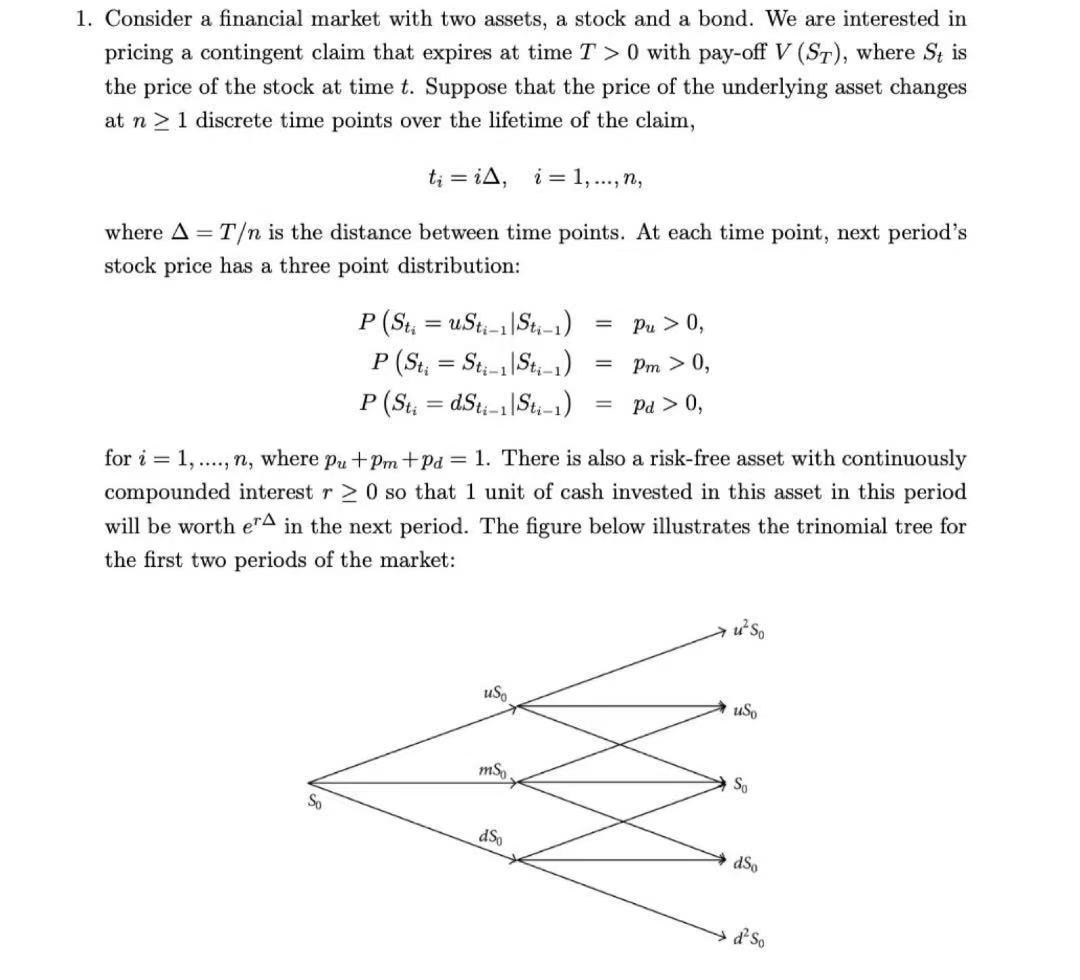
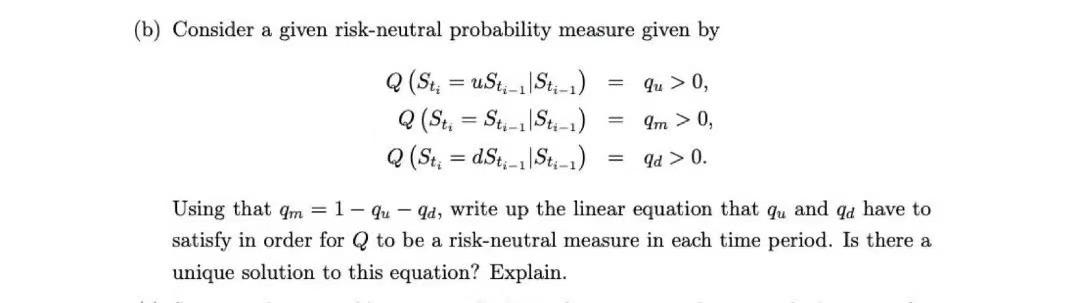
1. Consider a financial market with two assets, a stock and a bond. We are interested in pricing a contingent claim that expires at time T > 0 with pay-off V (ST), where St is the price of the stock at time t. Suppose that the price of the underlying asset changes at n > 1 discrete time points over the lifetime of the claim, t; = iA, i = 1, ..., n, where A=T is the distance between time points. At each time point, next period's stock price has a three point distribution: Pu > 0 P (Sti = uSt:-1 Sti-1) P (St; = St:-, Sti-1) P (St; = dSt-1 Sti-1) Pm > 0 Pd > 0 for i = 1,...., n, where pu +Pm+pa = 1. There is also a risk-free asset with continuously compounded interest r> 0 so that 1 unit of cash invested in this asset in this period will be worth era in the next period. The figure below illustrates the trinomial tree for the first two periods of the market: uso us, US ms So So ds. ds. ds. (b) Consider a given risk-neutral probability measure given by qu > 0 Q (St; = uSt;-1 St-1) Q (Sti = Sti-1 Sti-1) Q (St; = dSti-1 |St:-1) - Im > 0, ad > 0 Using that Im = 1- qu - 4d, write up the linear equation that qu and qd have to satisfy in order for Q to be a risk-neutral measure in each time period. Is there a unique solution to this equation? Explain. 1. Consider a financial market with two assets, a stock and a bond. We are interested in pricing a contingent claim that expires at time T > 0 with pay-off V (ST), where St is the price of the stock at time t. Suppose that the price of the underlying asset changes at n > 1 discrete time points over the lifetime of the claim, t; = iA, i = 1, ..., n, where A=T is the distance between time points. At each time point, next period's stock price has a three point distribution: Pu > 0 P (Sti = uSt:-1 Sti-1) P (St; = St:-, Sti-1) P (St; = dSt-1 Sti-1) Pm > 0 Pd > 0 for i = 1,...., n, where pu +Pm+pa = 1. There is also a risk-free asset with continuously compounded interest r> 0 so that 1 unit of cash invested in this asset in this period will be worth era in the next period. The figure below illustrates the trinomial tree for the first two periods of the market: uso us, US ms So So ds. ds. ds. (b) Consider a given risk-neutral probability measure given by qu > 0 Q (St; = uSt;-1 St-1) Q (Sti = Sti-1 Sti-1) Q (St; = dSti-1 |St:-1) - Im > 0, ad > 0 Using that Im = 1- qu - 4d, write up the linear equation that qu and qd have to satisfy in order for Q to be a risk-neutral measure in each time period. Is there a unique solution to this equation? Explain