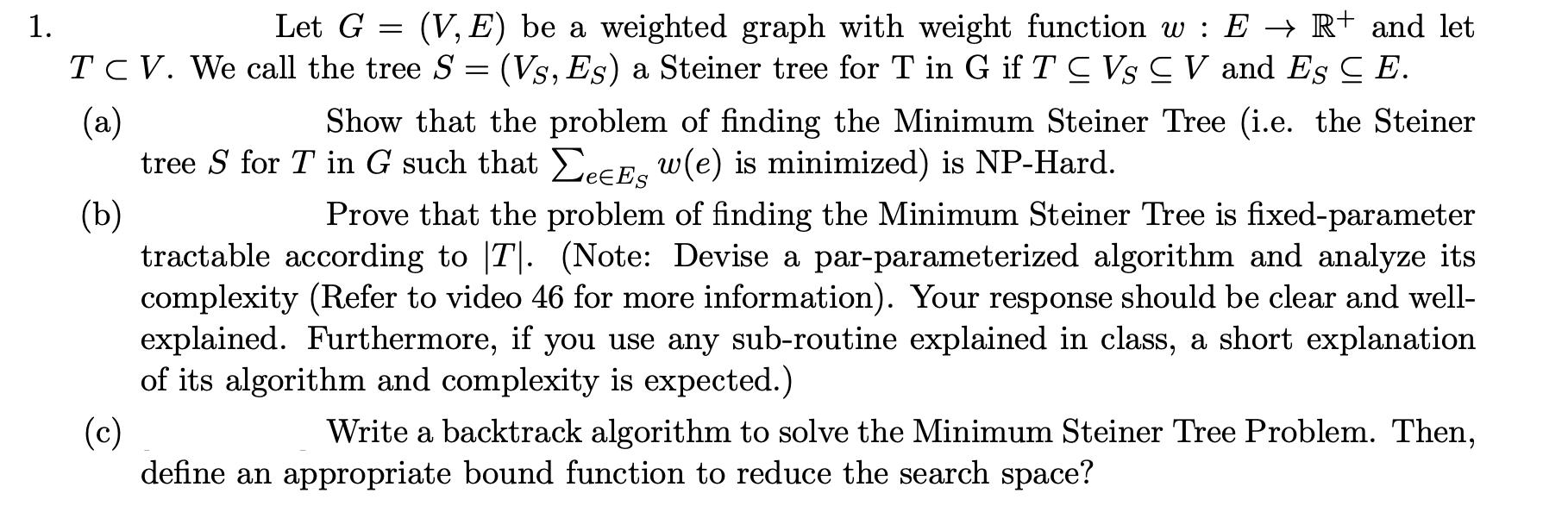
1. Let G = (V,E) be a weighted graph with weight function w: E R+ and let TC V. We call the tree S = (Vs, Es) a Steiner tree for T in G if TCVS CV and Es C E. (a) (b) (c) Show that the problem of finding the Minimum Steiner Tree (i.e. the Steiner tree S for T in G such that EES W(e) is minimized) is NP-Hard. Prove that the problem of finding the Minimum Steiner Tree is fixed-parameter tractable according to |T|. (Note: Devise a par-parameterized algorithm and analyze its complexity (Refer to video 46 for more information). Your response should be clear and well- explained. Furthermore, if you use any sub-routine explained in class, a short explanation. of its algorithm and complexity is expected.) Write a backtrack algorithm to solve the Minimum Steiner Tree Problem. Then, define an appropriate bound function to reduce the search space?