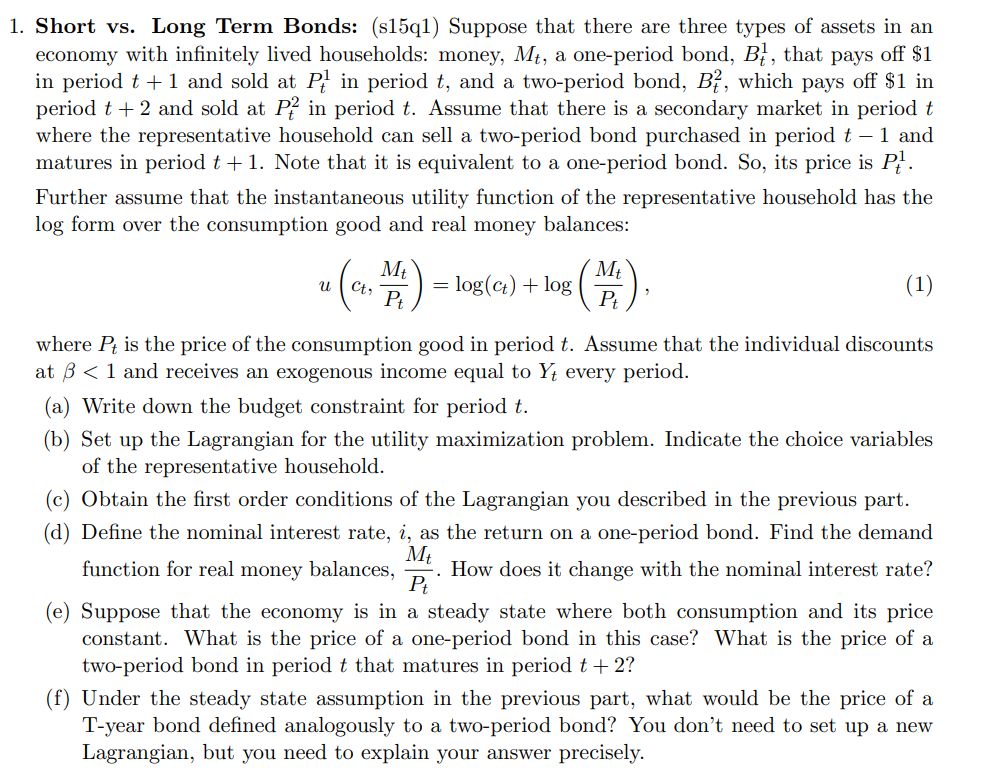
1. Short vs. Long Term Bonds: (s15q1) Suppose that there are three types of assets in an economy with infinitely lived households: money, Mt , a one-period bond, B1 t , that pays off $1 in period t + 1 and sold at P 1 t in period t, and a two-period bond, B2 t , which pays off $1 in period t + 2 and sold at P 2 t in period t. Assume that there is a secondary market in period t where the representative household can sell a two-period bond purchased in period t 1 and matures in period t + 1. Note that it is equivalent to a one-period bond. So, its price is P 1 t . Further assume that the instantaneous utility function of the representative household has the log form over the consumption good and real money balances: u ct , Mt Pt = log(ct) + log Mt Pt , (1) where Pt is the price of the consumption good in period t. Assume that the individual discounts at < 1 and receives an exogenous income equal to Yt every period. (a) Write down the budget constraint for period t. (b) Set up the Lagrangian for the utility maximization problem. Indicate the choice variables of the representative household. (c) Obtain the first order conditions of the Lagrangian you described in the previous part. (d) Define the nominal interest rate, i, as the return on a one-period bond. Find the demand function for real money balances, Mt Pt . How does it change with the nominal interest rate? (e) Suppose that the economy is in a steady state where both consumption and its price constant. What is the price of a one-period bond in this case? What is the price of a two-period bond in period t that matures in period t + 2? (f) Under the steady state assumption in the previous part, what would be the price of a T-year bond defined analogously to a two-period bond? You dont need to set up a new Lagrangian, but you need to explain your answer precisely

Short vs. Long Term Bonds: (s15q1) Suppose that there are three types of assets in an economy with infinitely lived households: money, Mt, a one-period bond, Bt1, that pays off $1 in period t+1 and sold at Pt1 in period t, and a two-period bond, Bt2, which pays off $1 in period t+2 and sold at Pt2 in period t. Assume that there is a secondary market in period t where the representative household can sell a two-period bond purchased in period t1 and matures in period t+1. Note that it is equivalent to a one-period bond. So, its price is Pt1. Further assume that the instantaneous utility function of the representative household has the log form over the consumption good and real money balances: u(ct,PtMt)=log(ct)+log(PtMt) where Pt is the price of the consumption good in period t. Assume that the individual discounts at <1 precisely