Answered step by step
Verified Expert Solution
Question
1 Approved Answer
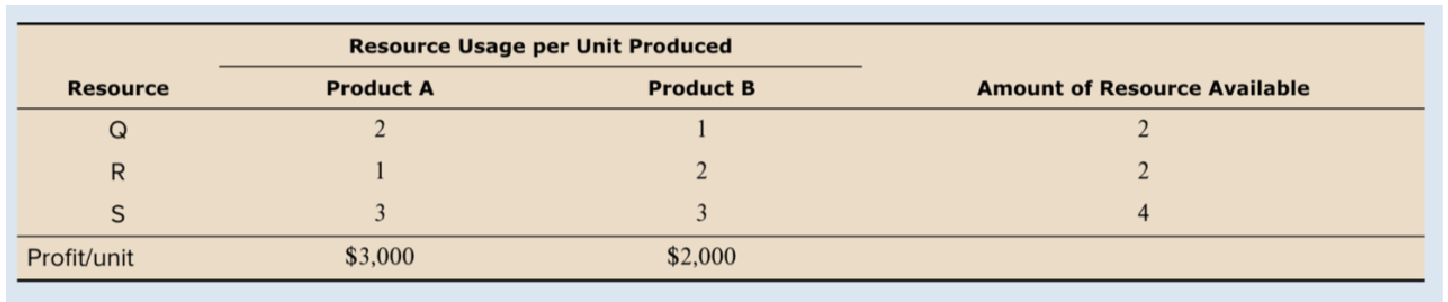
1 . The following table summarizes the key facts about two products, A and B , and the resources, Q , R , and S
The following table summarizes the key facts about two products, A and
B and the resources, Q R and S required to produce them.
The company needs to decide how many units of product A and product B to produce
for maximizing profit.
a Formulate the linear programming model algebraically.
b Formulate and solve a linear programming model for this problem on a
spreadsheet.
The Oak Works is a familyowned business that makes handcrafted dining
room tables and chairs. They obtain the oak from a local tree farm, which ships them
pounds of oak each month. Each table uses pounds of oak while each chair
uses pounds of oak. The family builds all the furniture itself and has hours of
labor available each month. Each table or chair requires six hours of labor. Each table
nets Oak Works $ in profit, while each chair nets $ in profit. Since chairs are
often sold with the tables, they want to produce at least twice as many chairs as tables.
The Oak Works would like to decide how many tables and chairs to produce so as to
maximize profit.
a Formulate the linear programming model algebraically.
b Formulate and solve a linear programming model for this problem on a
spreadsheet.
Weenies and Buns is a food processing plant that manufactures buns and
frankfurters for hot dogs. They grind their own flour for the buns at a maximum rate of
pounds per week. Each bun requires pound of flour. They currently have a
contract with Pigland, Inc., which specifies that a delivery of pounds of pork product
is delivered every Monday. Each frankfurter requires pound of pork product. All the
other ingredients in the buns and frankfurters are in plentiful supply. Finally, the labor
force at Weenies and Buns consists of five employees working full time hours per
week each Each bun requires two minutes of labor, and each frankfurter requires three
minutes of labor. Each bun yields a profit of $ and each frankfurter yields a profit of
$
Weenies and Buns would like to know how many buns and how many frankfurters they
should produce each week so as to achieve the highest possible profit.
a Formulate the linear programming model algebraically.
b Formulate and solve a linear programming model for this problem on a
spreadsheet.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


