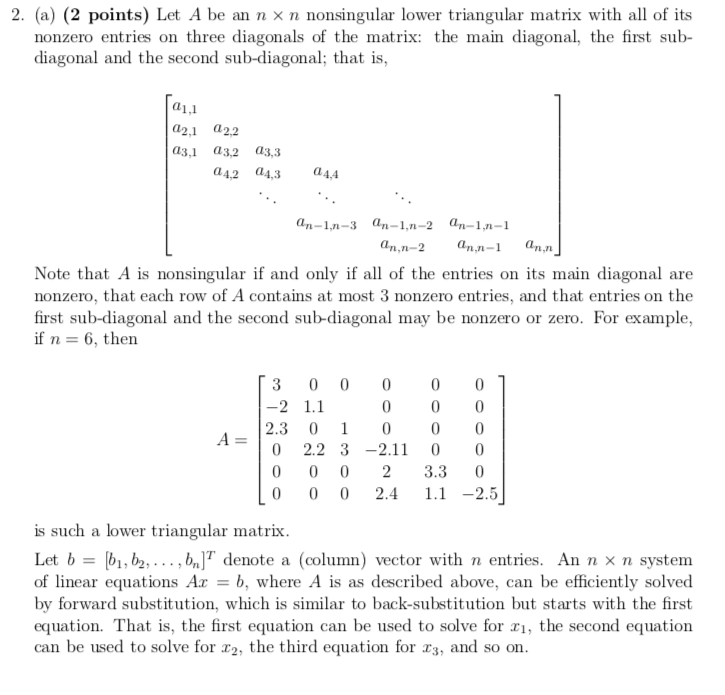

2. (a) (2 points) Let A be an n x n nonsingular lower triangular matrix with all of its nonzero entries on three diagonals of the matrix: the main diagonal, the first sub- diagonal and the second sub-diagonal; that is, 2,1 a22 3,1 a3,2 a3,3 a4.2 44,3 14.,4 an-1,n-3 an-1,n-2 An-1,n-1 dn,n-2 In.a-1 In Note that A is nonsingular if and only if all of the entries on its main diagonal are nonzero, that each row of A contains at most 3 nonzero entries, and that entries on the first sub-diagonal and the second sub-diagonal may be nonzero or zero. For example, if n 6, then 2 1.1 2.3 0 1 0 0 2.2 3 -2.11 0 0 0 0 0 2 3.3 0 0 0 02.4 1-2.5 is such a lower triangular matrix Let bb,bb denote a (column) vector with n entries. An n x n system of linear equations Ab, where A is as described above, can be efficiently solved by forward substitution, which is similar to back-substitution but starts with the first equation. That is, the first equation can be used to solve for x, the second equation can be used to solve for 2, the third equation for 3, and so on 2. (a) (2 points) Let A be an n x n nonsingular lower triangular matrix with all of its nonzero entries on three diagonals of the matrix: the main diagonal, the first sub- diagonal and the second sub-diagonal; that is, 2,1 a22 3,1 a3,2 a3,3 a4.2 44,3 14.,4 an-1,n-3 an-1,n-2 An-1,n-1 dn,n-2 In.a-1 In Note that A is nonsingular if and only if all of the entries on its main diagonal are nonzero, that each row of A contains at most 3 nonzero entries, and that entries on the first sub-diagonal and the second sub-diagonal may be nonzero or zero. For example, if n 6, then 2 1.1 2.3 0 1 0 0 2.2 3 -2.11 0 0 0 0 0 2 3.3 0 0 0 02.4 1-2.5 is such a lower triangular matrix Let bb,bb denote a (column) vector with n entries. An n x n system of linear equations Ab, where A is as described above, can be efficiently solved by forward substitution, which is similar to back-substitution but starts with the first equation. That is, the first equation can be used to solve for x, the second equation can be used to solve for 2, the third equation for 3, and so on








