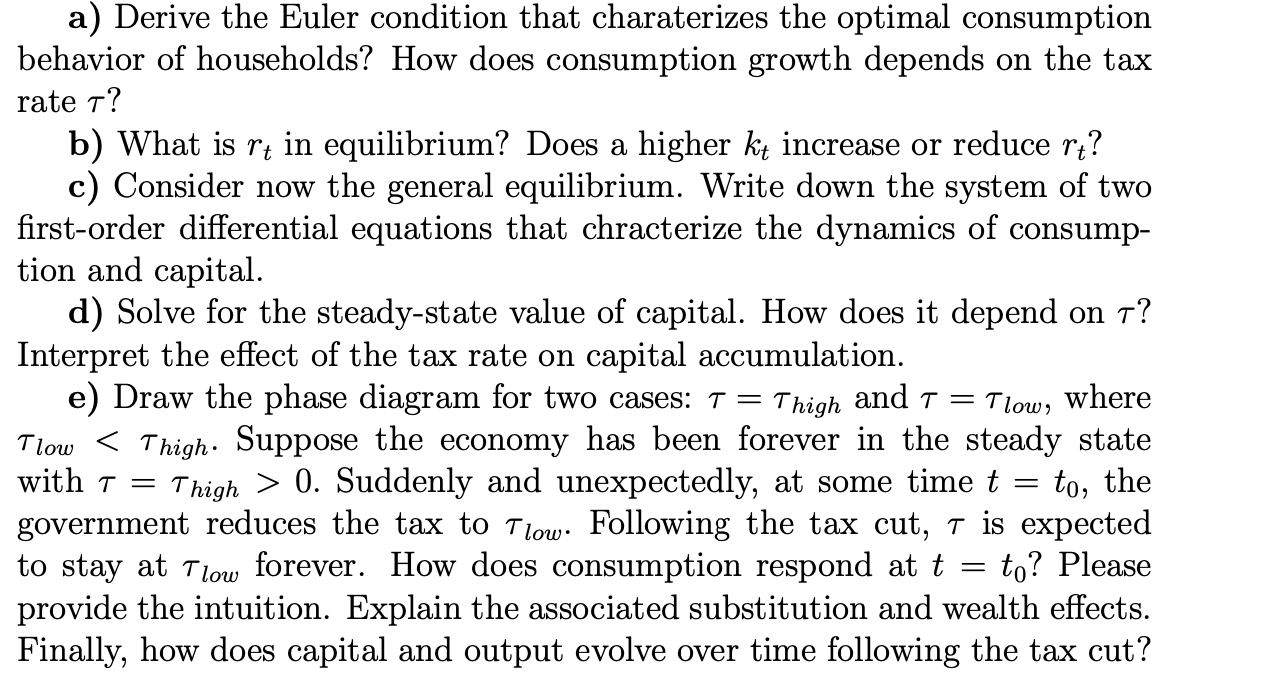
2 Neoclassical growth model Consider the neoclassical growth model, in discrete time. Assume that there is no population growth and no exogenous technological change; and normalize the size of the population and labor to 1. We examine decentralized competitive allocations. We introduce government in the model. The only role of the government is to redistribute income (there is no government spending). The government imposes a proportional tax on household income and uses the collected taxes to finance a lump-sum transfer to each household. Denote the time-invariant tax rate with T E (0, 1) and the transfer with Tt. The budget of the representative household is thus a tit = (1 - 1) (rtkt + wt) + Tt, (1) where C denotes consumption, it investment, kt capital, rt the interest rate, and w the wage rate. The capital stock accumulates according to kt+1 = it + (1 )kt, where 8 (0,1). The household's lifetime utility is given by c- U= s'u(Q)dt, u(c) = 1 - 0 where Be (0,1) is the discount factor and a > 0 is the elasticity of in- tertemporal substitution. The household mximizes (3) subject to (1) and (2), taking w; and rt, as well as Tt and T, as given. Firms are perfectly competitive and maximize profits. The technology is Cobb-Douglas. Output at time t is thus Yt = = f(kt) = ki where kt denotes the capital sock, yt denotes output or income, and a (0, 1). Note that, in equilibrium, yt = rtkt + Wt. Finally, the government budget requires that the tax revenue covers the cost of transfer, so T4 = T(r+kt + wt) = Tyt. a) Derive the Euler condition that charaterizes the optimal consumption behavior of households? How does consumption growth depends on the tax rate T? b) What is rt in equilibrium? Does a higher kt increase or reduce rt? c) Consider now the general equilibrium. Write down the system of two first-order differential equations that chracterize the dynamics of consump- tion and capital. d) Solve for the steady-state value of capital. How does it depend on t? Interpret the effect of the tax rate on capital accumulation. e) Draw the phase diagram for two cases: T = Thigh and t = Tlow Thigh. Suppose the economy has been forever in the steady state with t = Thigh > 0. Suddenly and unexpectedly, at some time t government reduces the tax to Tlow. Following the tax cut, T is expected to stay at Tlow forever. How does consumption respond at t to? Please provide the intuition. Explain the associated substitution and wealth effects. Finally, how does capital and output evolve over time following the tax cut? Tlow, where to, the = 2 Neoclassical growth model Consider the neoclassical growth model, in discrete time. Assume that there is no population growth and no exogenous technological change; and normalize the size of the population and labor to 1. We examine decentralized competitive allocations. We introduce government in the model. The only role of the government is to redistribute income (there is no government spending). The government imposes a proportional tax on household income and uses the collected taxes to finance a lump-sum transfer to each household. Denote the time-invariant tax rate with T E (0, 1) and the transfer with Tt. The budget of the representative household is thus a tit = (1 - 1) (rtkt + wt) + Tt, (1) where C denotes consumption, it investment, kt capital, rt the interest rate, and w the wage rate. The capital stock accumulates according to kt+1 = it + (1 )kt, where 8 (0,1). The household's lifetime utility is given by c- U= s'u(Q)dt, u(c) = 1 - 0 where Be (0,1) is the discount factor and a > 0 is the elasticity of in- tertemporal substitution. The household mximizes (3) subject to (1) and (2), taking w; and rt, as well as Tt and T, as given. Firms are perfectly competitive and maximize profits. The technology is Cobb-Douglas. Output at time t is thus Yt = = f(kt) = ki where kt denotes the capital sock, yt denotes output or income, and a (0, 1). Note that, in equilibrium, yt = rtkt + Wt. Finally, the government budget requires that the tax revenue covers the cost of transfer, so T4 = T(r+kt + wt) = Tyt. a) Derive the Euler condition that charaterizes the optimal consumption behavior of households? How does consumption growth depends on the tax rate T? b) What is rt in equilibrium? Does a higher kt increase or reduce rt? c) Consider now the general equilibrium. Write down the system of two first-order differential equations that chracterize the dynamics of consump- tion and capital. d) Solve for the steady-state value of capital. How does it depend on t? Interpret the effect of the tax rate on capital accumulation. e) Draw the phase diagram for two cases: T = Thigh and t = Tlow Thigh. Suppose the economy has been forever in the steady state with t = Thigh > 0. Suddenly and unexpectedly, at some time t government reduces the tax to Tlow. Following the tax cut, T is expected to stay at Tlow forever. How does consumption respond at t to? Please provide the intuition. Explain the associated substitution and wealth effects. Finally, how does capital and output evolve over time following the tax cut? Tlow, where to, the =