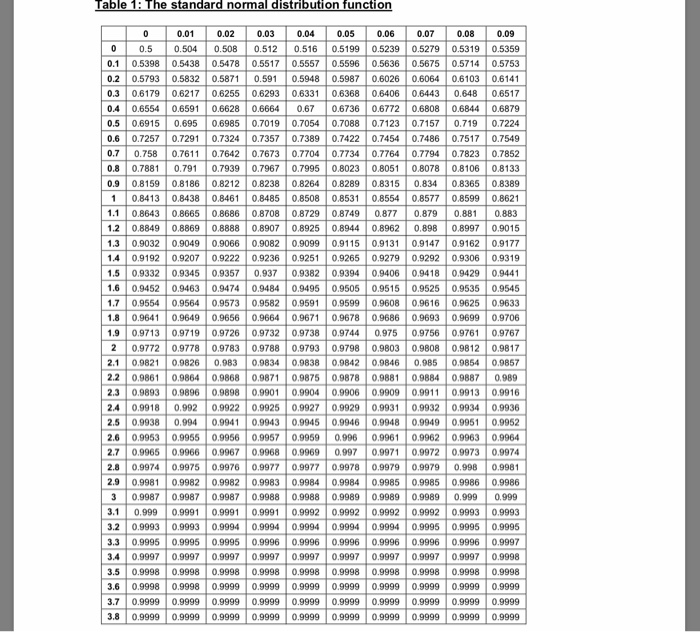
2 Suppose that a bond with maturity value m = 100 pays a coupon of c = 3.5 for two years (t = 2). Assume that the yield is y = 4.1%. Find: a) The price of the bond. [2 marks] b) Macauley Duration. [2 marks] Refer to the bond described above as bond 1, and assume that another bond (bond 2) is also available with m = 100, c = 3.65, 1 = 8, y = 4.4%. c) A company has signed a contract to pay 10m to a client in six years' time. In what combination should the company purchase the two bonds in order to eliminate interest rate risk? [3 marks) Consider C grade bonds with average cumulative default rate 19.1 for year 4 and 22.53 for year 5. d) For these bonds, what is the 1-year hazard rate for the 5th year? [3 marks) To fund an expansion in its operations Company X has just issued 20-year zero coupon bonds with a total face value of 195 million, taking its total asset value to 925 million. e) Explain how the value of the bonds can be expressed in terms of a European put option. [2 marks] f) Calculate the fair price of a holding of company bonds with face value of 100 using Black-Scholes model, given that the price of a 20-year zero- coupon government bond is 65.7047. Assume that the annualized volatility of the company's asset's over 20-year period is 27%. [3 marks] g) Estimate the risk-neutral probability of default on the company's bond. [1 mark] h) Suppose that the stock price at time zero is SO = 84. The continuously compounded risk free rate is 1.7%, and that a European call option written on S with strike price 80 and time to expiry T = 1 year has delta of 0.645 and trades for 12.2887. Find the implied volatility of the stock to the nearest 1%. [4 marks) Table 1: The standard normal distribution function 0 0.01 0 0.5 0.504 0.1 0.5398 0.5438 0.2 0.5793 0.5832 0.3 0.6179 0.6217 0.4 0.6554 0.6591 0.5 0.6915 0.695 0.6 0.7257 0.7291 0.7 0.758 0.7611 0.8 0.7881 0.791 0.9 0.8159 0.8186 1 0.8413 0.8438 1.1 0.8643 0.8665 1.2 0.8849 0.8869 1.3 0.9032 0.9049 1.4 0.9192 0.9207 1.5 0.9332 0.9345 1.6 0.9452 0.9463 1.7 0.9554 0.9564 1.8 0.9641 0.9649 1.9 0.97130.9719 2 0.9772 0.9778 2.1 0.9821 0.9826 2.2 0.9861 0.9864 2.3 0.9893 0.9896 2.4 0.9918 0.992 2.5 0.9938 0.994 2.6 0.9953 0.9955 2.7 0.9965 0.9966 2.8 0.9974 0.9975 2.9 0.9981 0.9982 3 0.9987 0.9987 3.1 0.999 0.9991 3.2 0.9993 0.9993 3.3 0.9995 0.9995 3.4 0.9997 0.9997 3.5 0.9998 0.9998 3.6 0.9998 0.9998 3.7 0.9999 0.9999 3.8 0.9999 0.9999 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.508 0.512 0.516 0.5199 0.5239 0.5279 0.5319 0.5359 0.5478 0.5517 0.5557 0.5596 0.5636 0.5675 0.5714 0.5753 0.5871 0.591 0.5948 0.5987 0.6026 0.6064 0.6103 0.6141 0.6255 0.6293 0.6331 0.6368 0.6406 0.6443 0.648 0.6517 0.6628 0.6664 0.67 0.6736 0.6772 0.6808 0.6844 0.6879 0.6985 0.7019 0.7054 0.7088 0.7123 0.7157 0.719 0.7224 0.7324 0.7357 0.7389 0.7422 0.7454 0.7486 0.7517 0.7549 0.7642 0.7673 0.7704 0.7734 0.7764 0.7794 0.78230.7852 0.7939 0.7967 0.7995 0.8023 0.8051 0.8078 0.8106 0.8133 0.8212 0.8238 0.8264 0.8289 0.8315 0.834 0.8365 0.8389 0.8461 0.8485 0.8508 0.8531 0.8554 0.8577 0.8599 0.8621 0.8686 0.8708 0.8729 0.8749 0.877 0.879 0.881 0.883 0.8888 0.8907 0.8925 0.8944 0.8962 0.898 0.8997 0.9015 0.9066 0.9082 0.9099 0.9115 0.9131 0.9147 0.9162 0.9177 0.9222 0.9236 0.9251 0.9265 0.9279 0.9292 0.9306 0.9319 0.9357 0.937 0.93820.9394 0.9406 0.9418 0.9429 0.9441 0.9474 0.9484 0.9495 0.9505 0.9515 0.9525 0.9535 0.9545 0.9573 0.9582 0.9591 0.9599 0.9608 0.9616 0.9625 0.9633 0.9656 0.9664 0.9671 0.9678 0.9686 0.9693 0.9699 0.9706 0.9726 0.9732 0.9738 0.9744 0.975 0.9756 0.9761 0.9767 0.9783 0.9788 0.9793 0.9798 0.9803 0.9808 0.98120.9817 0.983 0.9834 0.9838 0.9842 0.9846 0.985 0.9854 0.9857 0.9868 0.9871 0.9875 0.9878 0.9881 0.9884 0.9887 0.989 0.9898 0.9901 0.9904 0.9906 0.9909 0.99110.99130.9916 0.9922 0.9925 0.9927 0.9929 0.9931 0.9932 0.9934 0.9936 0.9941 0.9943 0.9945 0.9946 0.9948 0.9949 0.9951 0.9952 0.9956 0.9957 0.9959 0.996 0.9961 0.9962 0.99630.9964 0.9967 0.9968 0.9969 0.997 0.9971 0.9972 0.9973 0.9974 0.9976 0.9977 0.9977 0.9978 0.9979 0.9979 0.998 0.9981 0.9982 0.9983 0.9984 0.9984 0.9985 0.9985 0.9986 0.9986 0.9987 0.9988 0.9988 0.9989 0.9989 0.9989 0.999 0.999 0.9991 0.9991 0.9992 0.9992 0.9992 0.9992 0.9993 0.9993 0.9994 0.99940.99940.9994 0.9994 0.9995 0.9995 0.9995 0.9995 0.9996 0.9996 0.9996 0.9996 0.9996 0.9996 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 Distributions If Y - Lognormal (u,02), then the probability that Y is greater than cis: In) P(Y > c) = P(InY > Inc) =P ny"> lin 4) = P(z>lnc, 9) = 4("-dne) Bonds Price of a coupon-paying bond: C C+m p + ... + * 1+ y + (1+y)2 + (1+yjet (1+y) Macaulay Duration: D=- 1+ y op 1 1xc 2xc 3xC p J Pl(1+y) (1+y) (1+y) + + +...+ Tx(C+m) (1+y) Options The Black-Scholes formula for the value, c, at time t, of a European call option on a non-dividend-paying stock is: c = S0(d) - exp(-rt) Ko(d) p = exp(-ri)K(-d) - 5,0(-d) where: In S K + P + T 2 d, OVE S In 2 dz ori =d, -ovi and: Sy is the price of the underlying stock at time t, T(=T-t) is the time to expiry, r is the continuously compounded risk-free rate of interest, o is the price volatility of the underlying stock, K is the strike price, 0.) is the cumulative distribution function for a standard normal random variable. 2 Suppose that a bond with maturity value m = 100 pays a coupon of c = 3.5 for two years (t = 2). Assume that the yield is y = 4.1%. Find: a) The price of the bond. [2 marks] b) Macauley Duration. [2 marks] Refer to the bond described above as bond 1, and assume that another bond (bond 2) is also available with m = 100, c = 3.65, 1 = 8, y = 4.4%. c) A company has signed a contract to pay 10m to a client in six years' time. In what combination should the company purchase the two bonds in order to eliminate interest rate risk? [3 marks) Consider C grade bonds with average cumulative default rate 19.1 for year 4 and 22.53 for year 5. d) For these bonds, what is the 1-year hazard rate for the 5th year? [3 marks) To fund an expansion in its operations Company X has just issued 20-year zero coupon bonds with a total face value of 195 million, taking its total asset value to 925 million. e) Explain how the value of the bonds can be expressed in terms of a European put option. [2 marks] f) Calculate the fair price of a holding of company bonds with face value of 100 using Black-Scholes model, given that the price of a 20-year zero- coupon government bond is 65.7047. Assume that the annualized volatility of the company's asset's over 20-year period is 27%. [3 marks] g) Estimate the risk-neutral probability of default on the company's bond. [1 mark] h) Suppose that the stock price at time zero is SO = 84. The continuously compounded risk free rate is 1.7%, and that a European call option written on S with strike price 80 and time to expiry T = 1 year has delta of 0.645 and trades for 12.2887. Find the implied volatility of the stock to the nearest 1%. [4 marks) Table 1: The standard normal distribution function 0 0.01 0 0.5 0.504 0.1 0.5398 0.5438 0.2 0.5793 0.5832 0.3 0.6179 0.6217 0.4 0.6554 0.6591 0.5 0.6915 0.695 0.6 0.7257 0.7291 0.7 0.758 0.7611 0.8 0.7881 0.791 0.9 0.8159 0.8186 1 0.8413 0.8438 1.1 0.8643 0.8665 1.2 0.8849 0.8869 1.3 0.9032 0.9049 1.4 0.9192 0.9207 1.5 0.9332 0.9345 1.6 0.9452 0.9463 1.7 0.9554 0.9564 1.8 0.9641 0.9649 1.9 0.97130.9719 2 0.9772 0.9778 2.1 0.9821 0.9826 2.2 0.9861 0.9864 2.3 0.9893 0.9896 2.4 0.9918 0.992 2.5 0.9938 0.994 2.6 0.9953 0.9955 2.7 0.9965 0.9966 2.8 0.9974 0.9975 2.9 0.9981 0.9982 3 0.9987 0.9987 3.1 0.999 0.9991 3.2 0.9993 0.9993 3.3 0.9995 0.9995 3.4 0.9997 0.9997 3.5 0.9998 0.9998 3.6 0.9998 0.9998 3.7 0.9999 0.9999 3.8 0.9999 0.9999 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.508 0.512 0.516 0.5199 0.5239 0.5279 0.5319 0.5359 0.5478 0.5517 0.5557 0.5596 0.5636 0.5675 0.5714 0.5753 0.5871 0.591 0.5948 0.5987 0.6026 0.6064 0.6103 0.6141 0.6255 0.6293 0.6331 0.6368 0.6406 0.6443 0.648 0.6517 0.6628 0.6664 0.67 0.6736 0.6772 0.6808 0.6844 0.6879 0.6985 0.7019 0.7054 0.7088 0.7123 0.7157 0.719 0.7224 0.7324 0.7357 0.7389 0.7422 0.7454 0.7486 0.7517 0.7549 0.7642 0.7673 0.7704 0.7734 0.7764 0.7794 0.78230.7852 0.7939 0.7967 0.7995 0.8023 0.8051 0.8078 0.8106 0.8133 0.8212 0.8238 0.8264 0.8289 0.8315 0.834 0.8365 0.8389 0.8461 0.8485 0.8508 0.8531 0.8554 0.8577 0.8599 0.8621 0.8686 0.8708 0.8729 0.8749 0.877 0.879 0.881 0.883 0.8888 0.8907 0.8925 0.8944 0.8962 0.898 0.8997 0.9015 0.9066 0.9082 0.9099 0.9115 0.9131 0.9147 0.9162 0.9177 0.9222 0.9236 0.9251 0.9265 0.9279 0.9292 0.9306 0.9319 0.9357 0.937 0.93820.9394 0.9406 0.9418 0.9429 0.9441 0.9474 0.9484 0.9495 0.9505 0.9515 0.9525 0.9535 0.9545 0.9573 0.9582 0.9591 0.9599 0.9608 0.9616 0.9625 0.9633 0.9656 0.9664 0.9671 0.9678 0.9686 0.9693 0.9699 0.9706 0.9726 0.9732 0.9738 0.9744 0.975 0.9756 0.9761 0.9767 0.9783 0.9788 0.9793 0.9798 0.9803 0.9808 0.98120.9817 0.983 0.9834 0.9838 0.9842 0.9846 0.985 0.9854 0.9857 0.9868 0.9871 0.9875 0.9878 0.9881 0.9884 0.9887 0.989 0.9898 0.9901 0.9904 0.9906 0.9909 0.99110.99130.9916 0.9922 0.9925 0.9927 0.9929 0.9931 0.9932 0.9934 0.9936 0.9941 0.9943 0.9945 0.9946 0.9948 0.9949 0.9951 0.9952 0.9956 0.9957 0.9959 0.996 0.9961 0.9962 0.99630.9964 0.9967 0.9968 0.9969 0.997 0.9971 0.9972 0.9973 0.9974 0.9976 0.9977 0.9977 0.9978 0.9979 0.9979 0.998 0.9981 0.9982 0.9983 0.9984 0.9984 0.9985 0.9985 0.9986 0.9986 0.9987 0.9988 0.9988 0.9989 0.9989 0.9989 0.999 0.999 0.9991 0.9991 0.9992 0.9992 0.9992 0.9992 0.9993 0.9993 0.9994 0.99940.99940.9994 0.9994 0.9995 0.9995 0.9995 0.9995 0.9996 0.9996 0.9996 0.9996 0.9996 0.9996 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9998 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 0.9999 Distributions If Y - Lognormal (u,02), then the probability that Y is greater than cis: In) P(Y > c) = P(InY > Inc) =P ny"> lin 4) = P(z>lnc, 9) = 4("-dne) Bonds Price of a coupon-paying bond: C C+m p + ... + * 1+ y + (1+y)2 + (1+yjet (1+y) Macaulay Duration: D=- 1+ y op 1 1xc 2xc 3xC p J Pl(1+y) (1+y) (1+y) + + +...+ Tx(C+m) (1+y) Options The Black-Scholes formula for the value, c, at time t, of a European call option on a non-dividend-paying stock is: c = S0(d) - exp(-rt) Ko(d) p = exp(-ri)K(-d) - 5,0(-d) where: In S K + P + T 2 d, OVE S In 2 dz ori =d, -ovi and: Sy is the price of the underlying stock at time t, T(=T-t) is the time to expiry, r is the continuously compounded risk-free rate of interest, o is the price volatility of the underlying stock, K is the strike price, 0.) is the cumulative distribution function for a standard normal random variable