Question: 2.1 Minimizing a quadratic function and the curse of dimensionality Consider the simple quadratic function g(w) = ww (2.33) whose minimum is always at

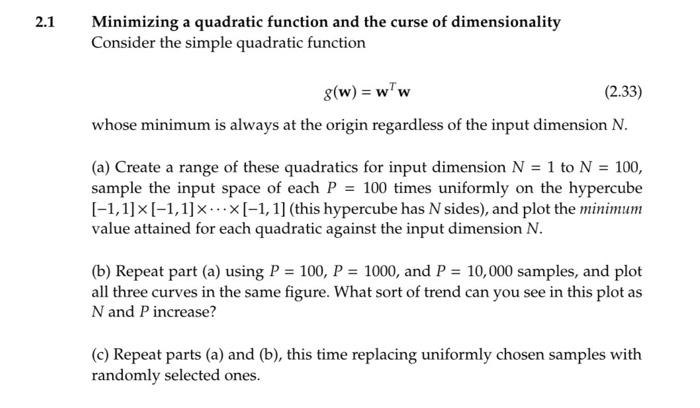
2.1 Minimizing a quadratic function and the curse of dimensionality Consider the simple quadratic function

2.1 Minimizing a quadratic function and the curse of dimensionality Consider the simple quadratic function g(w) = ww (2.33) whose minimum is always at the origin regardless of the input dimension N. (a) Create a range of these quadratics for input dimension N = 1 to N = 100, sample the input space of each P = 100 times uniformly on the hypercube [1,1]x[1,1]xx [-1, 1] (this hypercube has N sides), and plot the minimum value attained for each quadratic against the input dimension N. (b) Repeat part (a) using P = 100, P = 1000, and P = 10,000 samples, and plot all three curves in the same figure. What sort of trend can you see in this plot as N and P increase? (c) Repeat parts (a) and (b), this time replacing uniformly chosen samples with randomly selected ones.

Step by Step Solution

★★★★★

3.29 Rating (143 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Programming Questions!

Let f(x)= (1 - x if x 0 2x 1 if x

Managing Scope Changes Case Study Scope changes on a project can occur regardless of how well the project is planned or executed. Scope changes can be the result of something that was omitted during...

Planning is one of the most important management functions in any business. A front office managers first step in planning should involve determine the departments goals. Planning also includes...

1 2 x+2y= 10 2x - y = 5 x - y = 3 2x + y = 12 3 3x - 4y = 5 6x-4y = 2 4 3x - y = 1 5x + y = 7 5 2x - 7y = 1 2x + 3y = 11 6 2x-3y = 7 2x + 3y = 1 7 2x = 3y-14 2y = x+8 8 2(2x4) + y = 3 -x+2y= 4 9 y...

Math\t107-6381\t-\tQuiz\t#4\t-\tSchultz\t-\tDue\tFebruary\t21,\t2016\t-\tpage\t1\tof 3 Follow\tthese\tdirections\tcarefully. This\tquiz\tis\tdue\tby\t11:59\tEastern\ttime\ton\tFebruary\t21,\t2016. o...

ML in a nutshell Optimization, and machine learning, are intimately connected. At a very coarse level, ML works as follows. First, you come up somehow with a very complicated model y = M(x, 0), which...

Give Correct ANSWERS Human-Computer Interaction (a) If you had been one of the original inventors of the WIMP interface, and engineers on the technical team had been sceptical about the advantages...

1) Find all real solutions of: 4 2 2 x + x =3 2 x 4 + x23=0 4 2 2 2 x 2 x +3 x 3=0 x ( 21)=0 2 x 2 ( x 21)+ 3 ( 2 x2 +3 ) ( x 21 ) =0 ( 2 x2 +3 ) =0 2 2 x =3 3 x 2= 2 3 x= 2 3 x= i 2 2) Given the...

The new line character is utilized solely as the last person in each message. On association with the server, a client can possibly (I) question the situation with a client by sending the client's...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

Suppose that instead of having a rights issue of new stock at 4, Pandora decided to make a general cash offer at 4. Would existing shareholders still be just as well off? Explain.

Consider a binomial experiment with n = 20 and p = .4. Calculate P(x 10) using each of these methods: a. Table 1 in Appendix I b. The normal approximation to the binomial probability distribution

06.01-PR054 Packaging equipment for Xi Cling Wrap costs $60,000 and is expected to result in end-of-year net savings of $23,000 per year for 3 years. The equipment will have a market value of $10,000...

1. Which of the following is a correct match? (a) McClellandERG theory (b) Skinnerreinforcement theory (c) Vroomequity theory (d) Lockeexpectancy theory 2. In Herzbergs two-factor theory, base pay is...

The partnership of Telliher, Bachra, and Lang has experienced operating losses for three consecutive years. The partners, who have shared profits and losses in the ratio of Thea Telliher, 60 percent,...

The inexperienced driver of an all-wheel-drive car applies too much throttle as he attempts to accelerate from rest up the slippery 10-percent incline. The result is wheel spin at all four tires,...

a. Convert the heading control for the UFSS vehicle shown on the back endpapers (Johnson, 1980) into a digitally controlled system. b. Find the closed-loop pulse transfer function, T(z), if T = 0.1...

Critics of grocery club cards give examples of card-member prices being equal to the regular product price at stores without customer loyalty programs. In other words, customers who want to get food...

All of the following are ways that large companies attempt to mitigate the principal-agent problem EXCEPT bya. paying management bonuses that are tied to company performance.b. creating a company...

In upcoming chapters, we will often assume that the average cost curve is U-shaped. A: Indicate for each statement below whether you believe that the description of the firms situation would lead to...

In this exercise we review some basics of attitudes toward risk when tastes are state-independent and, in part B, we also verify some of the numbers that appear in the graphs of part A of the chapter...

In Gamble 1 you have a 99% chance of winning a trip to Venice and a 1% chance of winning tickets to a movie about Venice; and in Gamble 2, you have a 99% of winning the same trip to Venice and a 1%...

A study by Rikkers et al. (1978) involved a prospective randomized surgical trial that compared cirrhotic patients who had bled from use of either a nonselective shunt (a standard operation) or a...

The data set for this problem derives from the posture measurement study described in the main body of this chapter. Here we consider the data on shoulder flexion (SF) for 19 subjects that were each...

A study by Holder, Plikaytis, and Carlone (1996) compared two laboratory protocols designed to measure antibody levels in an enzyme-linked immunosorbent assay (ELISA) for Streptococcus pneumoniae....