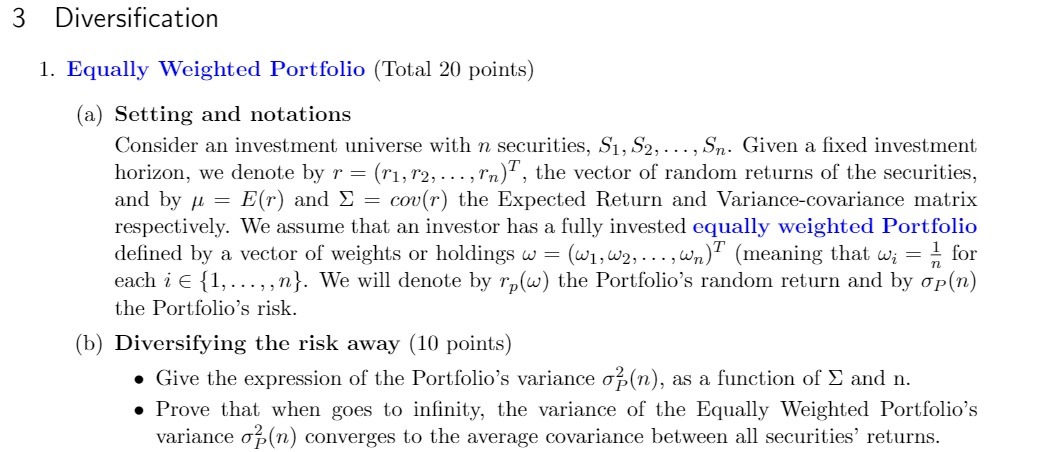
3 Diversification 1. Equally Weighted Portfolio (Total 20 points) (a) Setting and notations Consider an investment universe with n securities, S1, S2, ..., Sn. Given a fixed investment horizon, we denote by r = (r1, T2,,rn), the vector of random returns of the securities, and by = E(r) and = cov(r) the Expected Return and Variance-covariance matrix respectively. We assume that an investor has a fully invested equally weighted Portfolio defined by a vector of weights or holdings w = (w1, W2,..., wn) (meaning that w;= for each i {1,...,, n}. We will denote by rp (w) the Portfolio's random return and by p(n) the Portfolio's risk. (b) Diversifying the risk away (10 points) Give the expression of the Portfolio's variance (n), as a function of and n. Prove that when goes to infinity, the variance of the Equally Weighted Portfolio's variance (n) converges to the average covariance between all securities' returns.