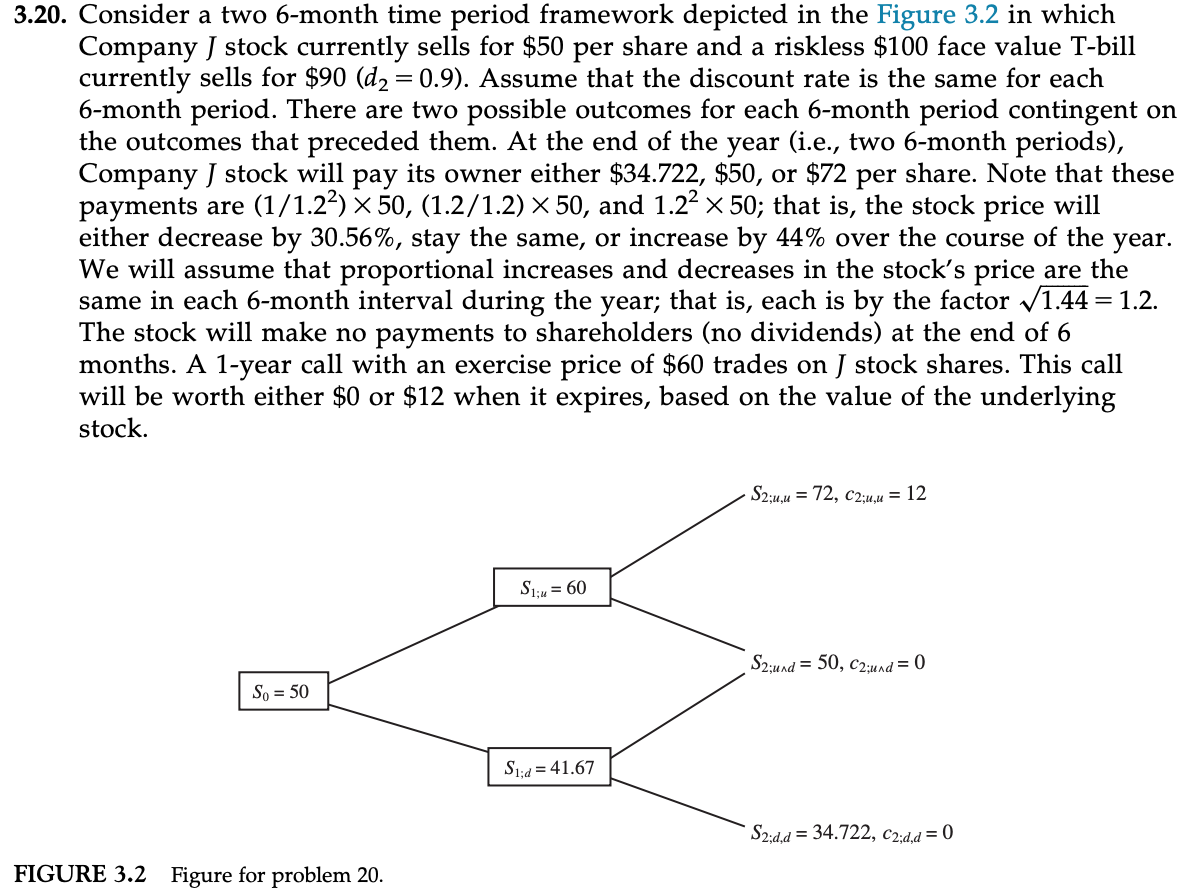
3.20. Consider a two 6-month time period framework depicted in the Figure 3.2 in which Company J stock currently sells for $50 per share and a riskless $100 face value T-bill currently sells for $90 (d2 = 0.9). Assume that the discount rate is the same for each 6-month period. There are two possible outcomes for each 6-month period contingent on the outcomes that preceded them. At the end of the year (i.e., two 6-month periods), Company J stock will pay its owner either $34.722, $50, or $72 per share. Note that these payments are (1/1.22) x 50, (1.2/1.2) X 50, and 1.22 x 50; that is, the stock price will either decrease by 30.56%, stay the same, or increase by 44% over the course of the year. We will assume that proportional increases and decreases in the stock's price are the same in each 6-month interval during the year; that is, each is by the factor 1.44 = 1.2. The stock will make no payments to shareholders (no dividends) at the end of 6 months. A 1-year call with an exercise price of $60 trades on J stock shares. This call will be worth either $0 or $12 when it expires, based on the value of the underlying stock. S2;u,u = 72, C2;u,u = 12 S1;u = 60 S2;und = 50, C2;und = 0 So = 50 S1:a = 41.67 S2;d,d = 34.722, C2;d,d = 0 FIGURE 3.2 Figure for problem 20. a. Write 1-year payoff vectors for the stock, T-bill, and the call. b. Assume that risk-neutral probabilities associated with upjumps are constant over the two 6-month intervals during the year in this framework. Are markets complete in this four- outcome two-period market? Why or why not? c. Assume that risk-neutral probabilities associated with downjumps are constant over the two 6-month intervals during the year as are riskless rates in this framework. What is the pure security price associated with each upjump? What is the pure security price associated with each downjump? d. From your answers to a, b, and c above, find risk-neutral probabilities for the first 6-month interval, again assuming that the 6-month riskless interest rate is r=0.0541. That is, find 90,1;d and 90,1;u e. What are the three two-period pure security prices 40,2;d,d, 40,2;und, and 40,2;u,u? f. What is the current value of the call? 3.20. Consider a two 6-month time period framework depicted in the Figure 3.2 in which Company J stock currently sells for $50 per share and a riskless $100 face value T-bill currently sells for $90 (d2 = 0.9). Assume that the discount rate is the same for each 6-month period. There are two possible outcomes for each 6-month period contingent on the outcomes that preceded them. At the end of the year (i.e., two 6-month periods), Company J stock will pay its owner either $34.722, $50, or $72 per share. Note that these payments are (1/1.22) x 50, (1.2/1.2) X 50, and 1.22 x 50; that is, the stock price will either decrease by 30.56%, stay the same, or increase by 44% over the course of the year. We will assume that proportional increases and decreases in the stock's price are the same in each 6-month interval during the year; that is, each is by the factor 1.44 = 1.2. The stock will make no payments to shareholders (no dividends) at the end of 6 months. A 1-year call with an exercise price of $60 trades on J stock shares. This call will be worth either $0 or $12 when it expires, based on the value of the underlying stock. S2;u,u = 72, C2;u,u = 12 S1;u = 60 S2;und = 50, C2;und = 0 So = 50 S1:a = 41.67 S2;d,d = 34.722, C2;d,d = 0 FIGURE 3.2 Figure for problem 20. a. Write 1-year payoff vectors for the stock, T-bill, and the call. b. Assume that risk-neutral probabilities associated with upjumps are constant over the two 6-month intervals during the year in this framework. Are markets complete in this four- outcome two-period market? Why or why not? c. Assume that risk-neutral probabilities associated with downjumps are constant over the two 6-month intervals during the year as are riskless rates in this framework. What is the pure security price associated with each upjump? What is the pure security price associated with each downjump? d. From your answers to a, b, and c above, find risk-neutral probabilities for the first 6-month interval, again assuming that the 6-month riskless interest rate is r=0.0541. That is, find 90,1;d and 90,1;u e. What are the three two-period pure security prices 40,2;d,d, 40,2;und, and 40,2;u,u? f. What is the current value of the call