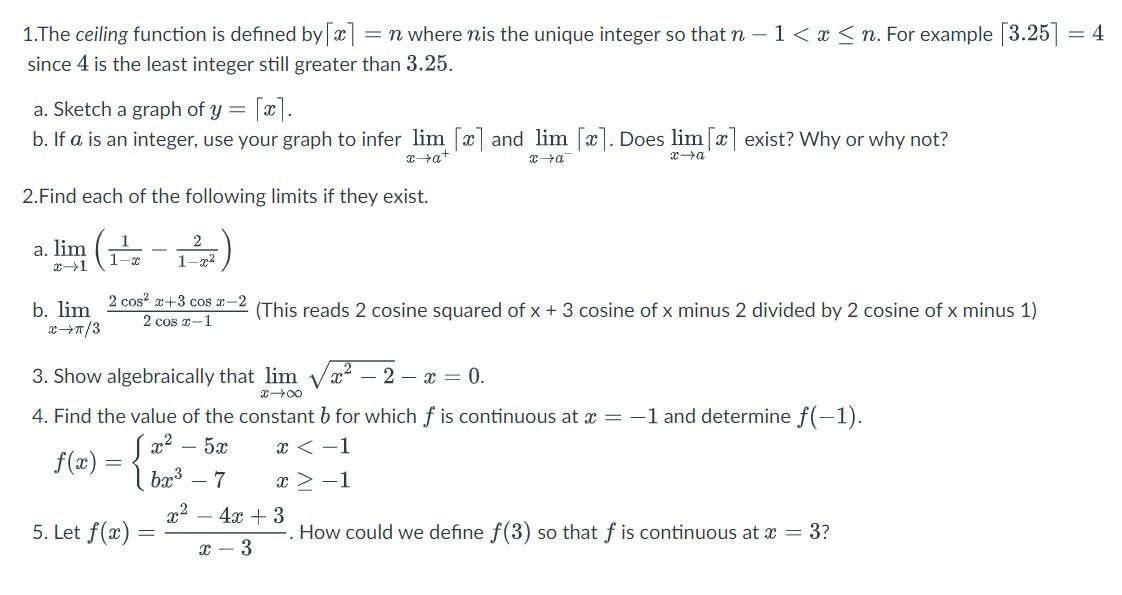
= 4 1.The ceiling function is defined by [x] = n where nis the unique integer so that n-1 < xn. For example [3.25] since 4 is the least integer still greater than 3.25. a. Sketch a graph of y = [x]. b. If a is an integer, use your graph to infer lim [x] and lim [x]. Does lim [x] exist? Why or why not? xa+ xa xa 2.Find each of the following limits if they exist. (18 a. lim x-1 b. lim xn/3 2 cos2x+3 cos x-2 2 cos x-1 2 1-x 3. Show algebraically that lim x 2 x = 0. 318 f(x) = = 4. Find the value of the constant b for which f is continuous at x = -1 and determine f(-1). x - 5x x < -1 bx x>-1 5. Let f(x)= (This reads 2 cosine squared of x + 3 cosine of x minus 2 divided by 2 cosine of x minus 1) - 7 - 4x + 3 X 3 -. How could we define f(3) so that f is continuous at x = 3?