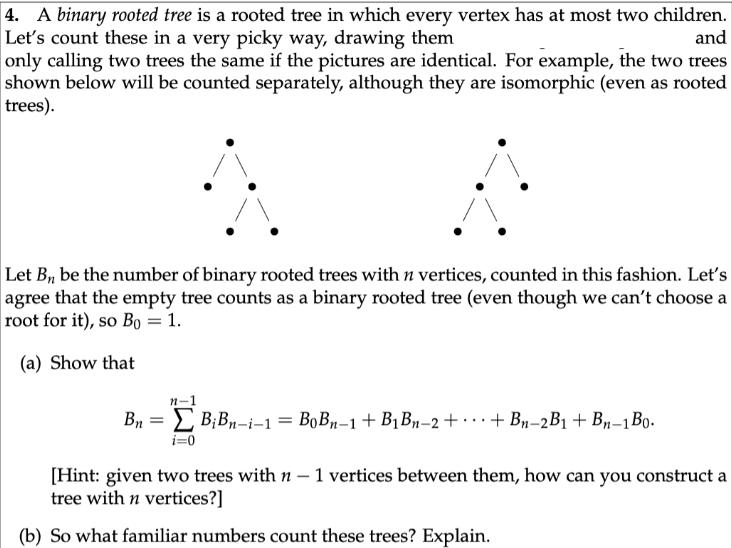
4. A binary rooted tree is a rooted tree in which every vertex has at most two children. Let's count these in a very picky way, drawing them and only calling two trees the same if the pictures are identical. For example, the two trees shown below will be counted separately, although they are isomorphic (even as rooted trees). Let B, be the number of binary rooted trees with n vertices, counted in this fashion. Let's agree that the empty tree counts as a binary rooted tree (even though we can't choose root for it), so Bo = 1. (a) Show that n-1 Bn = BiBn-i-1 = BoBn1 + BBn-2 + + Bn2B + Bn1B0. ... i=0 [Hint: given two trees with n - 1 vertices between them, how can you construct tree with n vertices?] (b) So what familiar numbers count these trees? Explain.