Answered step by step
Verified Expert Solution
Question
1 Approved Answer
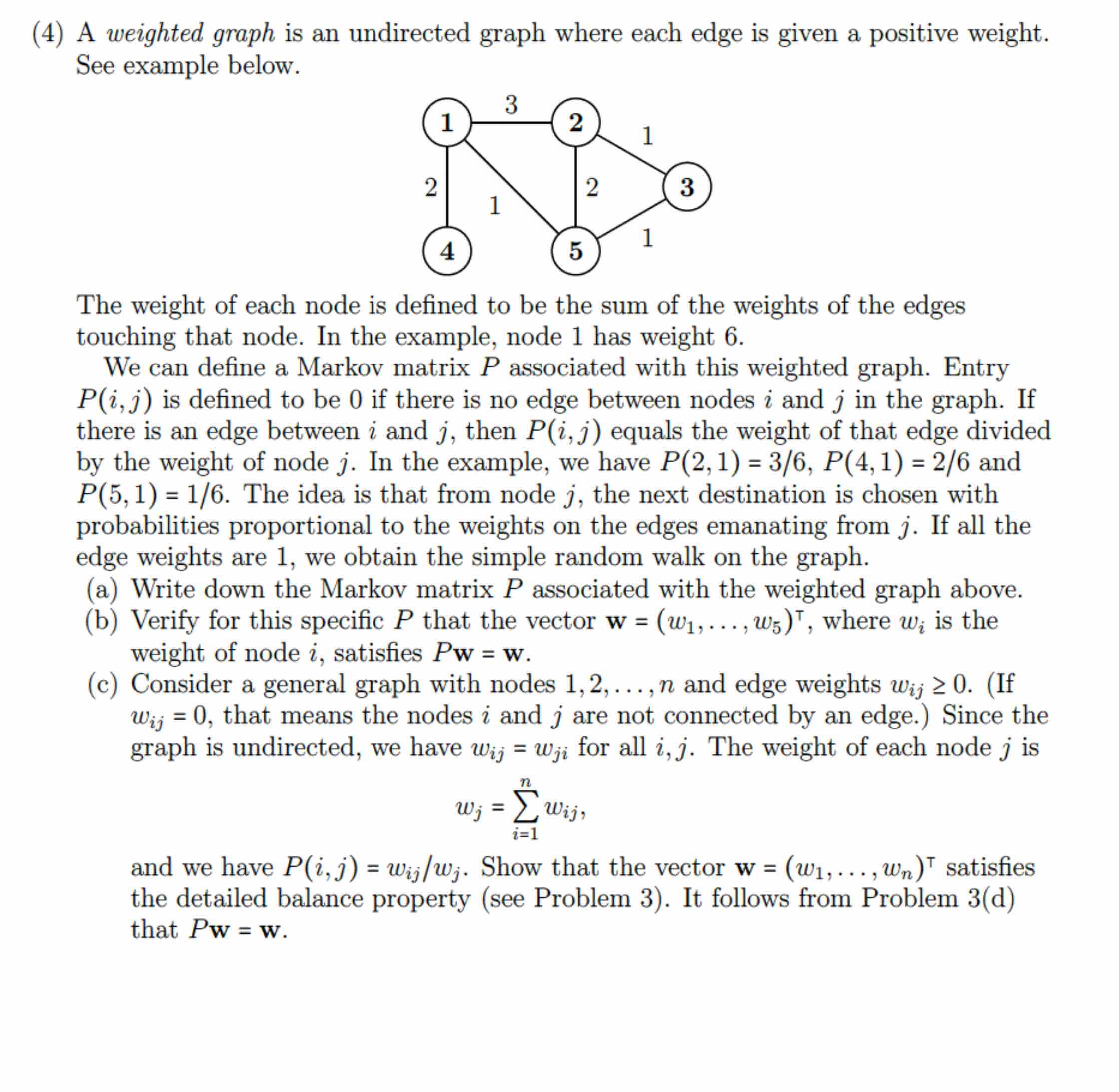
( 4 ) A weighted graph is an undirected graph where each edge is given a positive weight. See example below. The weight of each
A weighted graph is an undirected graph where each edge is given a positive weight.
See example below.
The weight of each node is defined to be the sum of the weights of the edges
touching that node. In the example, node has weight
We can define a Markov matrix associated with this weighted graph. Entry
is defined to be if there is no edge between nodes i and in the graph. If
there is an edge between i and then equals the weight of that edge divided
by the weight of node In the example, we have and
The idea is that from node the next destination is chosen with
probabilities proportional to the weights on the edges emanating from If all the
edge weights are we obtain the simple random walk on the graph.
a Write down the Markov matrix associated with the weighted graph above.
b Verify for this specific that the vector dots, where is the
weight of node satisfies
c Consider a general graph with nodes dots, and edge weights If
that means the nodes i and are not connected by an edge. Since the
graph is undirected, we have for all The weight of each node is
and we have Show that the vector dots, satisfies
the detailed balance property see Problem It follows from Problem d
that
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


