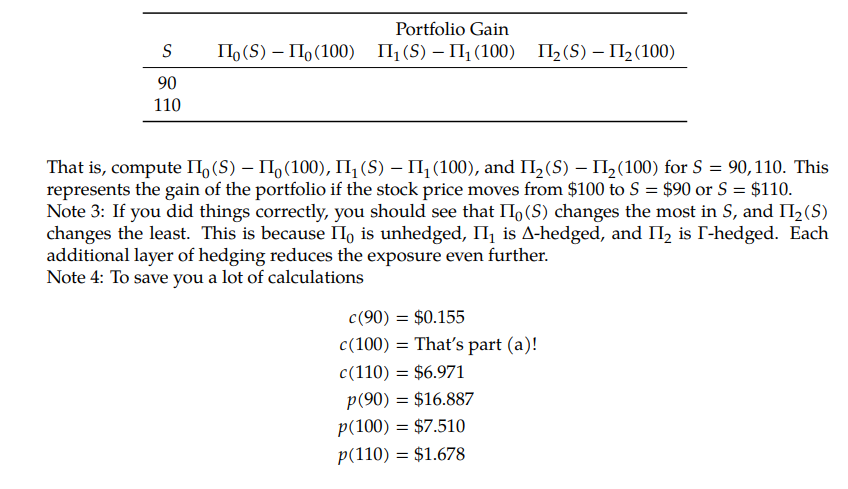
4 Black-Scholes-Merton and Hedging (30 points) Consider a stock with a price of 100 and annual volatility =0.2. Suppose that all interest rates are 1% p.a. with continuous compounding. For simplicity, suppose that all options are for 1 share of the underlying stock. 1 For this problem, use the 252 trading day convention. Suppose you have a position of 10 European call options with strike price 105 and time to expiration of 50 trading days. (a) (5 points) Compute the Black-Scholes-Merton price of the call option you hold, as well as the and . Leave your answers in terms of a single call option (don't multiply by 10 to get the portfolio values yet). (b) (5 points) Compute the (Black-Scholes-Merton) of this option. Briefly explain why 1. (c) (5 points) What position would you need to have in the stock to form a delta-neutral portfolio? (d) (5 points) Suppose you also have access to European put options with strike price 107 and time to expiration of 30 trading days. Find the (Black-Scholes-Merton) price, , and of this put option. (e) (5 points) What position would you need to have in the stock and the put option from part (d) to have a delta-neutral and gamma-neutral portfolio? (You should be solving two equations in two unknowns here.) (f) (5 points) Let's do some "scenario analysis" to check that this hedging strategy actually works. For notation, let 0(S) denote the value of the original portfolio of 10 call options when the stock price is S. Let 1(S) denote the value of the 10 call options plus the position in the stock you computed in part (c) when the stock price is S. Let 2(S) denote the value of the portfolio consisting of the 10 call options plus the positions in the stock and put options you calculated in part (e) when the stock price is S. Do not include any cash in these values. For example, if you found you needed to short 5 stocks (this is not the right answer) in (c), then 1(S)=10c(S)5S. Complete the following table: That is, compute 0(S)0(100),1(S)1(100), and 2(S)2(100) for S=90,110. This represents the gain of the portfolio if the stock price moves from $100 to S=$90 or S=$110. Note 3: If you did things correctly, you should see that 0(S) changes the most in S, and 2(S) changes the least. This is because 0 is unhedged, 1 is -hedged, and 2 is -hedged. Each additional layer of hedging reduces the exposure even further. Note 4: To save you a lot of calculations c(90)c(100)c(110)p(90)p(100)p(110)=$0.155=Thatspart(a)!=$6.971=$16.887=$7.510=$1.678 4 Black-Scholes-Merton and Hedging (30 points) Consider a stock with a price of 100 and annual volatility =0.2. Suppose that all interest rates are 1% p.a. with continuous compounding. For simplicity, suppose that all options are for 1 share of the underlying stock. 1 For this problem, use the 252 trading day convention. Suppose you have a position of 10 European call options with strike price 105 and time to expiration of 50 trading days. (a) (5 points) Compute the Black-Scholes-Merton price of the call option you hold, as well as the and . Leave your answers in terms of a single call option (don't multiply by 10 to get the portfolio values yet). (b) (5 points) Compute the (Black-Scholes-Merton) of this option. Briefly explain why 1. (c) (5 points) What position would you need to have in the stock to form a delta-neutral portfolio? (d) (5 points) Suppose you also have access to European put options with strike price 107 and time to expiration of 30 trading days. Find the (Black-Scholes-Merton) price, , and of this put option. (e) (5 points) What position would you need to have in the stock and the put option from part (d) to have a delta-neutral and gamma-neutral portfolio? (You should be solving two equations in two unknowns here.) (f) (5 points) Let's do some "scenario analysis" to check that this hedging strategy actually works. For notation, let 0(S) denote the value of the original portfolio of 10 call options when the stock price is S. Let 1(S) denote the value of the 10 call options plus the position in the stock you computed in part (c) when the stock price is S. Let 2(S) denote the value of the portfolio consisting of the 10 call options plus the positions in the stock and put options you calculated in part (e) when the stock price is S. Do not include any cash in these values. For example, if you found you needed to short 5 stocks (this is not the right answer) in (c), then 1(S)=10c(S)5S. Complete the following table: That is, compute 0(S)0(100),1(S)1(100), and 2(S)2(100) for S=90,110. This represents the gain of the portfolio if the stock price moves from $100 to S=$90 or S=$110. Note 3: If you did things correctly, you should see that 0(S) changes the most in S, and 2(S) changes the least. This is because 0 is unhedged, 1 is -hedged, and 2 is -hedged. Each additional layer of hedging reduces the exposure even further. Note 4: To save you a lot of calculations c(90)c(100)c(110)p(90)p(100)p(110)=$0.155=Thatspart(a)!=$6.971=$16.887=$7.510=$1.678