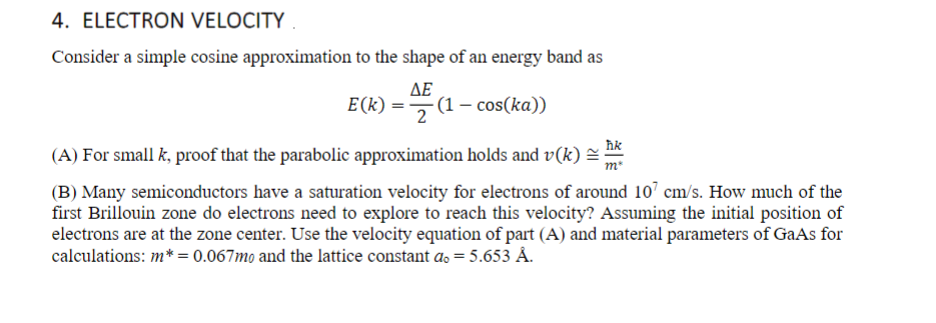
4. ELECTRON VELOCITY Consider a simple cosine approximation to the shape of an energy band as E(k) = (1 - cos(ka)) (A) For small k, proof that the parabolic approximation holds and v(k) hk m* (B) Many semiconductors have a saturation velocity for electrons of around 107 cm/s. How much of the first Brillouin zone do electrons need to explore to reach this velocity? Assuming the initial position of electrons are at the zone center. Use the velocity equation of part (A) and material parameters of GaAs for calculations: m* = 0.067mo and the lattice constant do = 5.653 .