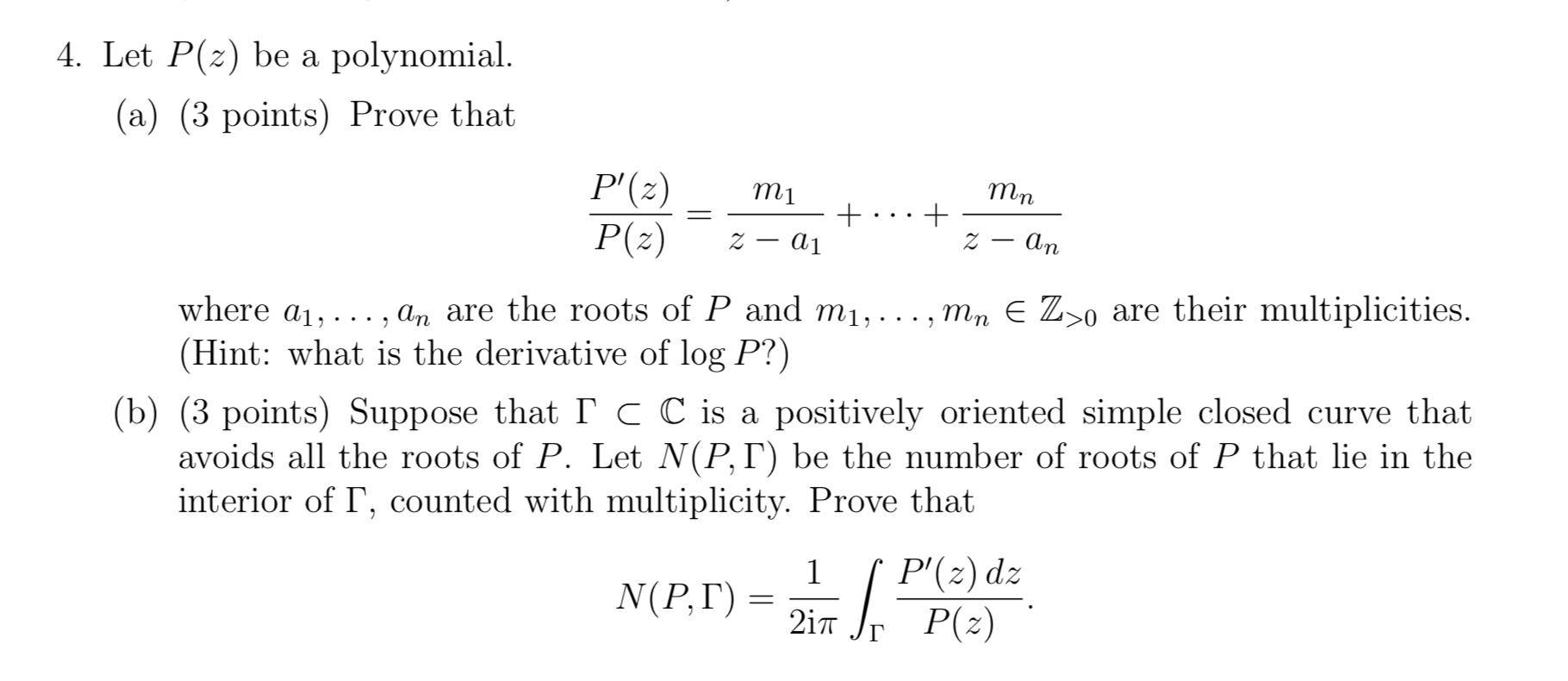
4. Let P(z) be a polynomial (a) (3 points) Prove that '() P(2) i m Z-an _ where a1,. . . , an are the roots of P and m1,. . . , mn E Z>0 are their multiplicities (Hint: what is the derivative of log P?) (b) (3 points) Suppose that Tc C is a positively oriented simple closed curve that avoids all the roots of P. Let N(P,T) be the number of roots of P that lie in the interior of I, counted with multiplicity. Prove that (2) dz (?) N(P.) 2i