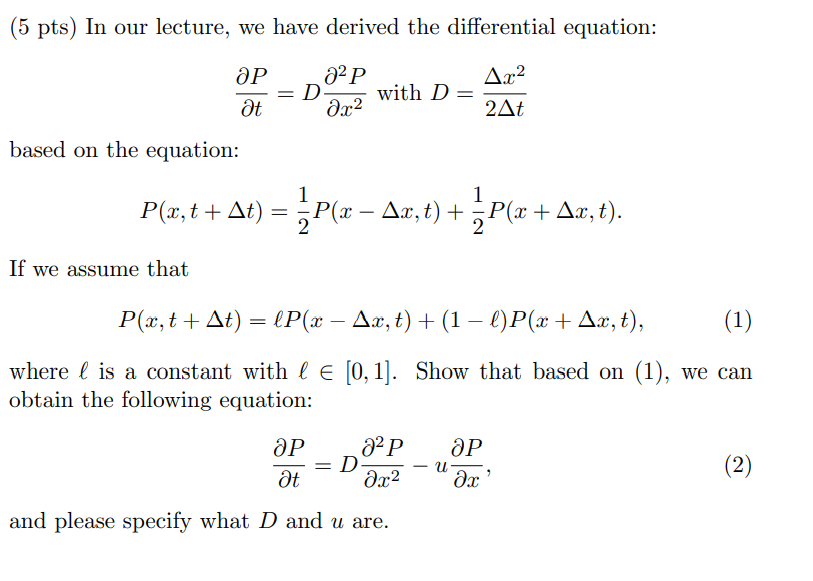
(5 pts) In our lecture, we have derived the differential equation: t a P Ax2 = D with D = 2 2At based on the equation: P(x,t+^t) = P(x x,t) + - Ax, t) + + P(x - P(x + x,t). If we assume that P(x,t+t) = lP(x Ax, t) + (1 l)P(x + x, t), - (1) where is a constant with l [0,1]. Show that based on (1), we can obtain the following equation: = D t a P 2 and please specify what D and u are. - (2)