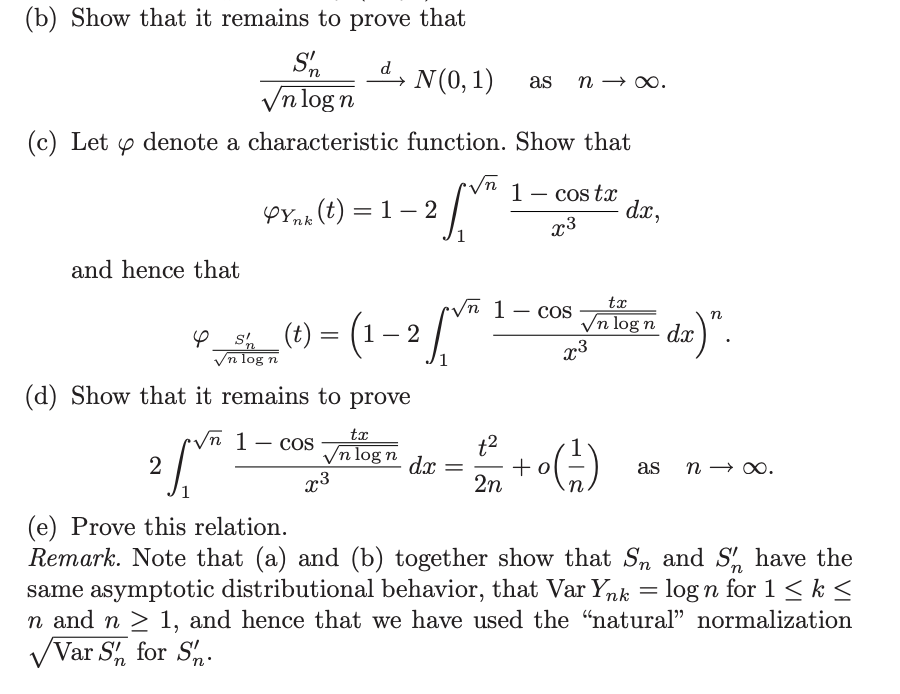50. The purpose of this problem is to show that one can obtain a (kind of) central limit theorem even if the summands have infinite variance (if the variance does not exist). A short introduction to the general topic of possible limit theorems for normalized sums without finite variance is given in Section 7.3. Let X1,X2, be independent random variables with the following symmetric Pareto distribution: fX(x)={x31,0,forx>1otherwise. 8 Problems 185 Set Sn=k=1nXk,n1. Show via the following steps that nlognSndN(0,1)asn (Note that we do not normalize by n as in the standard case.) Fix n and consider, for k=1,2,,n, the truncated random variables Ynk={Xk,0,whenXknotherwise and Znk={Xk,0,whenXk>n,otherwise, and note that Ynk+Znk=Xk. Further, set Sn=k=1nYnk and Sn= k=1nZnk (a) Show that EnlognSn0asn and conclude that nlognSn0in1-meanasn, and hence in probability (why?). (b) Show that it remains to prove that nlognSndN(0,1)asn (c) Let denote a characteristic function. Show that Ynk(t)=121nx31costxdx and hence that nlognSn(t)=(121nx31cosnlogntxdx)n. (d) Show that it remains to prove 21nx31cosnlogntxdx=2nt2+o(n1)asn. (e) Prove this relation. Remark. Note that (a) and (b) together show that Sn and Sn have the same asymptotic distributional behavior, that VarYnk=logn for 1k n and n1, and hence that we have used the "natural" normalization VarSn for Sn