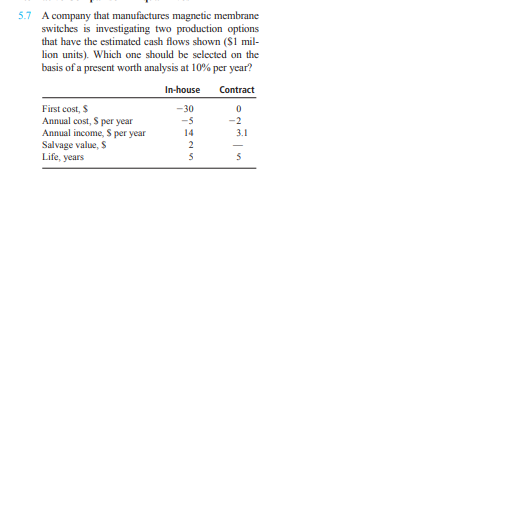
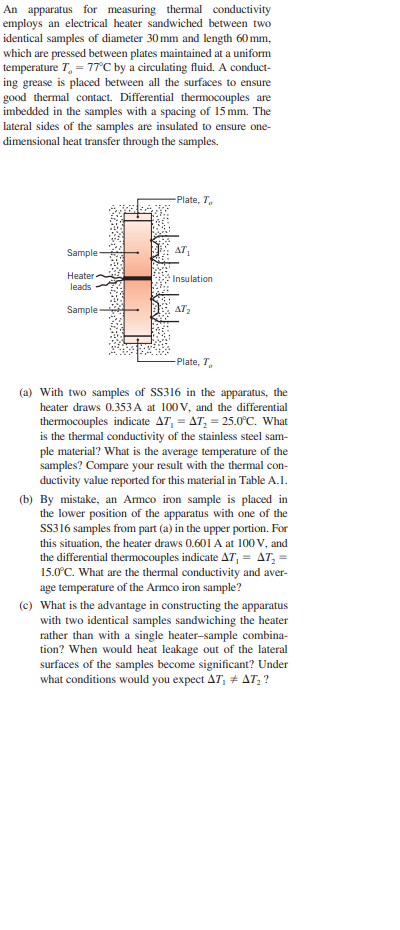
5.7 A company that manufactures magnetic membrane switches is investigating two production options that have the estimated cash flows shown ($1 mil- lion units). Which one should be selected on the basis of a present worth analysis at 10% per year? In-house Contract First cost, $ -30 Annual cost, $ per year -S Annual income, $ per year 14 Salvage value, $ Life, yearsThe tensile strength of silicone rubber is thought to be a function of curing temperature. A study was carried out in which samples of 12 specimens of the rubber were prepared using curing temperatures of 20 C and 45 C. The data below show the tensile strength values in megapascals. 20 C: 2.07 2.14 2.22 2.03 2.21 2.03 2.05 2.18 2.09 2.14 2.11 2.02 45"C: 2.52 2.15 2.49 2.03 2.37 2.05 1.99 2.42 2.08 2.42 2.29 2.01 (a) Show a dot plot of the data with both low and high temperature tensile strength values. (b) Compute sample mean tensile strength for both samples. (c) Does it appear as if curing temperature has an influence on tensile strength, based on the plot? Comment further. (d) Does anything else appear to be influenced by an increase in curing temperature? Explain.Twenty adult males between the ages of 30 and 40 participated in a study to evaluate the effect of a specific health regimen involving diet and exercise on the blood cholesterol. Ten were randomly selected to be a control group, and ten others were assigned to take part in the regimen as the treatment group for a period of 6 months. The following data show the reduction in cholesterol experienced for the time period for the 20 subjects: Control group: 7 3 -4 14 2 522 -795 Treatment group: -6 5 9 4 4 12 37 5 3 3 (a) Do a dot plot of the data for both groups on the same graph. (b) Compute the mean, median, and 10% trimmed mean for both groups. (c) Explain why the difference in means suggests one conclusion about the effect of the regimen while the difference in medians or trimmed means suggests a different conclusion.An apparatus for measuring thermal conductivity employs an electrical heater sandwiched between two identical samples of diameter 30 mm and length 60 mm, which are pressed between plates maintained at a uniform temperature 7, = 77'C by a circulating fluid. A conduct- ing grease is placed between all the surfaces to ensure good thermal contact. Differential thermocouples are imbedded in the samples with a spacing of 15 mm. The lateral sides of the samples are insulated to ensure one- dimensional heat transfer through the samples. Plate, To Sample AT Heater Insulation leads Sample -Plate, I. (a) With two samples of SS316 in the apparatus, the heater draws 0.353 A at 100 V, and the differential thermocouples indicate A7, = AT, = 25.0'C. What is the thermal conductivity of the stainless steel sam- ple material? What is the average temperature of the samples? Compare your result with the thermal con- ductivity value reported for this material in Table A.1. (b) By mistake, an Armco iron sample is placed in the lower position of the apparatus with one of the SS316 samples from part (a) in the upper portion. For this situation, the heater draws 0.601 A at 100 V, and the differential thermocouples indicate A7, = AT, = 15.0"C. What are the thermal conductivity and aver- age temperature of the Armco iron sample? (c) What is the advantage in constructing the apparatus with two identical samples sandwiching the heater rather than with a single heater-sample combina- tion? When would heat leakage out of the lateral surfaces of the samples become significant? Under what conditions would you expect AT, # AT, ?The waiting time, in hours, between successive speeders spotted by a radar unit is a continuous random variable with cumulative distribution function Find the probability of waiting less than 12 minutes between successive speeders (a) using the cumulative distribution function of X; (b) using the probability density function of X. Determine the value c so that each of the following functions can serve as a probability distribution of the discrete random variable X: (a) f(x) = c(x2 + 4), for x = 0, 1, 2, 3; (b) f(x) = c() (->), for x = 0, 1, 2.The probability distribution of X, the number of imperfections per 10 meters of a synthetic fabric in continuous rolls of uniform width, is given by 1 2 3 I(x) 0.41 0.37 0.16 0.05 0.01 Construct the cumulative distribution function of X. Let X denote the diameter of an armored electric cable and Y denote the diameter of the ceramic mold that makes the cable. Both X and Y are scaled so that they range between 0 and 1. Suppose that X and Y have the joint density f (z, y) = - 01/2)