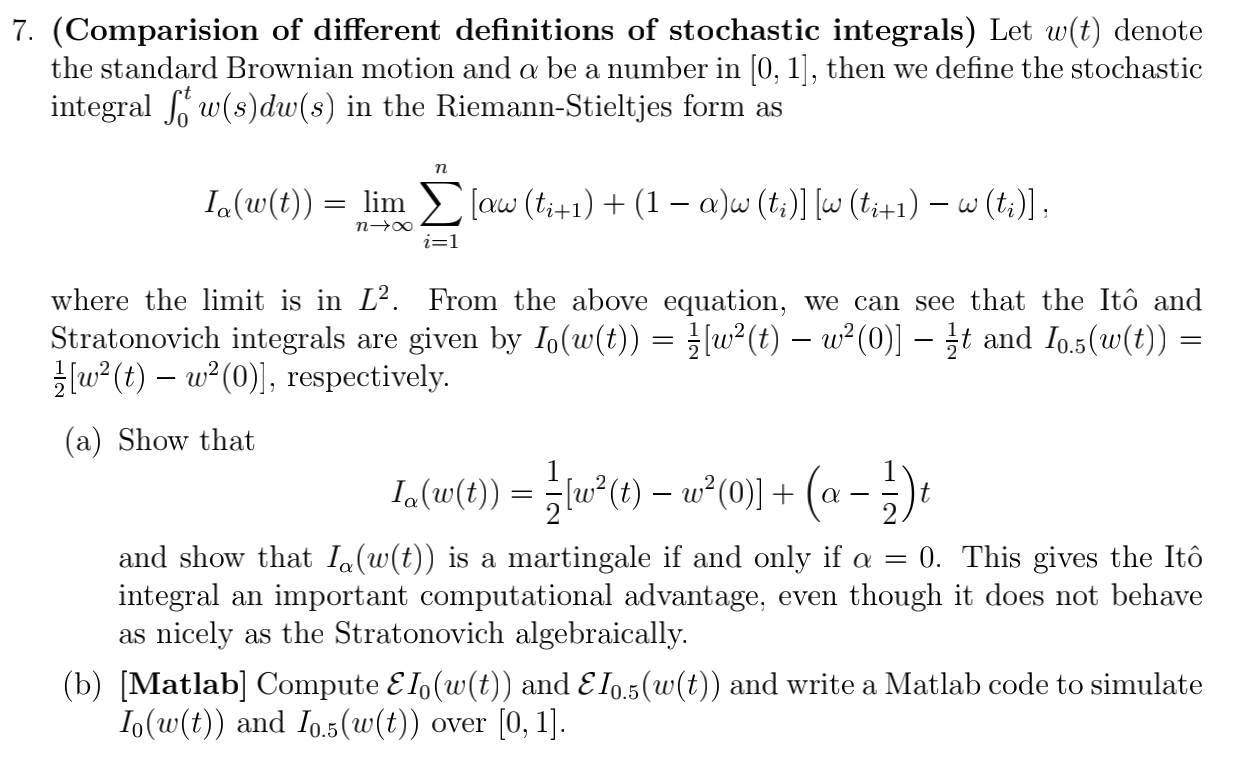
7. (Comparision of different definitions of stochastic integrals) Let w(t) denote the standard Brownian motion and a be a number in [0, 1], then we define the stochastic integral for w(s) dw(s) in the Riemann-Stieltjes form as n - Ia(w(t)) = lim [aw (ti+1) + (1 a)w (ti)] [w (ti+1) w (ti)]; NX i=1 where the limit is in L. From the above equation, we can see that the It and Stratonovich integrals are given by Io (w(t)) = [w(t) w(0)] and 10.5(w(t)) = [w(t) w(0)], respectively. (a) Show that Ia(w(t)) = = {w (t) w(0)] + (a - )t - and show that I (w(t)) is a martingale if and only if a = 0. This gives the It integral an important computational advantage, even though it does not behave as nicely as the Stratonovich algebraically. (b) [Matlab] Compute & I (w(t)) and 10.5(w(t)) and write a Matlab code to simulate Io(w(t)) and I0.5(w(t)) over [0, 1].