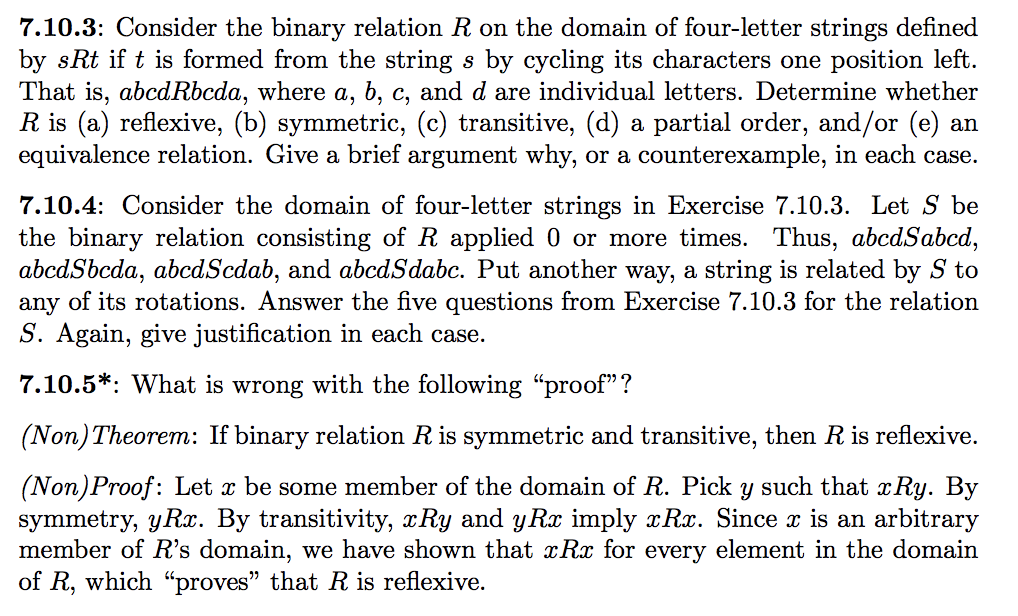
7.10.3: Consider the binary relation R on the domain of four-letter strings defined by sRt if t is formed from the string s by cycling its characters one position left That is, abcdRbcda, where a, b, c, and d are individual letters. Determine whether R is (a) reflexive, (b) symmetric, (c) transitive, (d) a partial order, and/or (e) an equivalence relation. Give a brief argument why, or a counterexample, in each case. 7.10.3. Let S be 7.10.4: Consider the domain of four-letter strings in Exercise the binary relation consisting of R applied 0 or more times. Thus, abcdSabcd abcdSbcda, abcdScdab, and abcdSdabc. Put another way, a string is related by S to any of its rotations. Answer the five questions from Exercise 7 S. Again, give justification in each case. .10.3 for the relation 7.10.5*: What is wrong with the following "proof"? (Non)Theorem: If binary relation R is symmetric and transitive, then R is reflexive. (Non)Proof: Let x be some member of the domain of R. Pick y such that xRy. By symmetry, yRx. By transitivity, xRy and yRx imply xRx. Since x is an arbitrary member of R's domain, we have shown that xRx for every element in the domain of R, which "proves" that R is reflexive. 7.10.3: Consider the binary relation R on the domain of four-letter strings defined by sRt if t is formed from the string s by cycling its characters one position left That is, abcdRbcda, where a, b, c, and d are individual letters. Determine whether R is (a) reflexive, (b) symmetric, (c) transitive, (d) a partial order, and/or (e) an equivalence relation. Give a brief argument why, or a counterexample, in each case. 7.10.3. Let S be 7.10.4: Consider the domain of four-letter strings in Exercise the binary relation consisting of R applied 0 or more times. Thus, abcdSabcd abcdSbcda, abcdScdab, and abcdSdabc. Put another way, a string is related by S to any of its rotations. Answer the five questions from Exercise 7 S. Again, give justification in each case. .10.3 for the relation 7.10.5*: What is wrong with the following "proof"? (Non)Theorem: If binary relation R is symmetric and transitive, then R is reflexive. (Non)Proof: Let x be some member of the domain of R. Pick y such that xRy. By symmetry, yRx. By transitivity, xRy and yRx imply xRx. Since x is an arbitrary member of R's domain, we have shown that xRx for every element in the domain of R, which "proves" that R is reflexive