Question: 8. An upper-triangular linear system has the form a11 a12 a13 a14 21 0 0221 023 a24 22 b 0 0 0 033 034

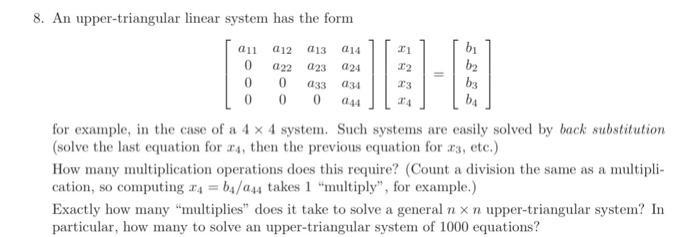
8. An upper-triangular linear system has the form a11 a12 a13 a14 21 0 0221 023 a24 22 b 0 0 0 033 034 00 23 b3 044 ba for example, in the case of a 4 x 4 system. Such systems are easily solved by back substitution (solve the last equation for 4, then the previous equation for x3, etc.) How many multiplication operations does this require? (Count a division the same as a multipli- cation, so computing x4 =b4/044 takes 1 "multiply", for example.) Exactly how many "multiplies" does it take to solve a general n x n upper-triangular system? In particular, how many to solve an upper-triangular system of 1000 equations?

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Programming Questions!

6 . Debt Policy a . State and explain M&M ' s Proposition I. b . State and explain M&M ' s Proposition II . c . Briefly describe the traditionalists position on capital structure. d . Draw a graph of...

Portray in words what transforms you would have to make to your execution to some degree (a) to accomplish this and remark on the benefits and detriments of this thought.You are approached to compose...

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

1. What velocity will a 300 kg snowmobile acquire if pushed from rest by a force of 6240 N [E] for 1.25 s? What average force will stop this snowmobile from moving at this speed in 1.25 s? (v = 26.0...

So you're the boss. Now what? Assume you have just been promoted to a managerial position. What key thoughts and actions would you reflect on in order to develop and maintain a highly productive...

Name three cognitive or cognitive-behavioral therapists. How are their views similar? How are their perspectives different?

Carter Corporation made sales of $900 million during 2016. Of this amount, Carter collected cash for $871 million. The company's cost of goods sold was $280 million, and all other expenses for the...

Prepare Form 1040 pg 1&2, Schedule A, B, and D pg 1 & 2. Form 1040 assume Janis is under age 65 line 40 - total Itemized Deductions from Schedule A tax on line 44 should be $19,799 -- see Schedule D...

As the accountant for Runson Moving Company, you are preparing the companys annual return, Form 940 and Schedule A. Use the following information to complete Form 940 and Schedule A on pages 5-40 to...

According to the lead author of the study, what is one reason wind companies should address shadow flicker? Question 5Answer a. To minimize aesthetic impacts on local scenery b. To avoid legal...

On January 1, 20X1, Porta Corporation purchased Swick Company's net assets and assigned goodwill of $80,100 to Reporting Division K. The following assets and liabilities are assigned to Reporting...

SARAD 09 (Photo courtesy of Caterpillar) A re-seller of mining machinery, located in Stratford, Ontario, calls a CIFFA Forwarder, inquiring about shipping a used Caterpillar Grader, to Vina del Mar,...

Report on the engagement activities that have occurred with your group during the term and share your personal/individual plans to continue interactions beyond the course. Produce either a text...

A short, cantilevered round peg is 40 mm in diameter and extends 10 mm from the wall. It is loaded, as shown, with a force F = 50 kN applied at the tip in the negative z direction, and a torque T=...

11. Todd fractured his wrist while he was Rollerblading. To relieve the pain, his doctor suggested that he take 500 mg of aspirin every 6 h. At this rate, only 26% of the aspirin remains in his body...

5 pts Question 12 Moody Corporation's bonds have a 15-year maturity, a 7.25% coupon paid semiannually, and a par value of $1.000. If the going market interest rate for bonds of similar risk and...

A handrail, which weighs 120 N and is 1.8 m long. was mounted to a wall adjacent to a small set of steps (Figure P4.26). The support at A has broken, and the rail has fallen about the loose bolt at 8...

Consider the boundary value problem - u"+ u = x, for 0 Answered: 1 week ago

Answer Exercise 7.5.24 for (a) The weighted norm ||(x, y)T|| = 2x2 + 3y2; (b) The norm based on (c) The norm based on Exercise 7.5.24 Minimize ||(2x - y, x + y)T||2 - 6x over all x, y, where || ||...

Explain why a matrix with a row of all zeros does not have an inverse.

A flat plate with a chord length L of 3.5 m, Try to estimate the thickness of the boundary layer at the rear edge of the slab (all laminar flow). ReL 105

If the velocity distribution functions of the boundary layer are and Try to calculate the displacement, momentum loss and energy loss thickness of the boundary layer. n Voo

If the velocity distribution functions of the boundary layer are and please calculate the displacement, momentum loss, and energy loss thickness of the boundary layer. 36 n Voo 8