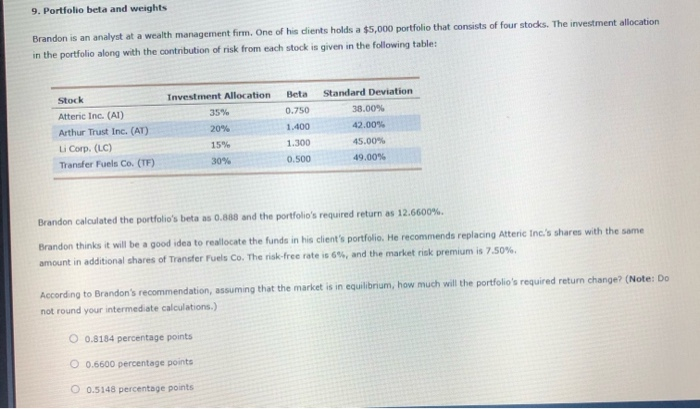
9. Portfolio beta and weights Brandon is an analyst at a wealth management firm. One of his dients holds a $5,000 portfolio that consists of four stocks. The investment allocation in the portfolio along with the contribution of risk from each stock is given in the following table: Standard Deviation 38.00% Investment Allocation 35% 20% 15% Stock Atteric Inc. (AI) Arthur Trust Inc. (AT) Li Corp. (LC) Transfer Fuel Co. (TF) Beta 0.750 1.400 1.300 0.500 42.00% 45.00% 49.00% 30% Brandon calculated the portfolio's beta as 0.888 and the portfolio's required return as 12.6600%. Brandon thinks it will be a good idea to reallocate the funds in his client's portfolio. He recommends replacing Atteric Inc.'s shares with the same amount in additional shares of Transfer Fuels Co. The risk-free rate is 6%, and the market risk premium is 7.50%. According to Brandon's recommendation, assuming that the market is in equilibrium, how much will the portfolio's required return change? (Note: Do not round your intermediate calculations.) O 0.8184 percentage points O 0.6600 percentage points O 0.5148 percentage points not round your intermediate calculations.) O 0.8184 percentage points O 0.6600 percentage points O 0.5148 percentage points O 0.7590 percentage points Analysts' estimates on expected returns from equity investments are based on several factors. These estimations also often indude subjective and judgmental factors, because different analysts interpret data in different ways. Suppose, based on the earnings consensus of stock analysts, Brandon expects a return of 13.50% from the portfolio with the new weights. Does he think that the required return as compared to expected returns is undervalued, overvalued, or fairly valued? O Undervalued O Overvalued O Fairly valued Suppose instead of replacing Attenc Inc.'s stock with Transfer Fuels Co.'s stock, Brandon considers replacing Atteric Inc.'s stock with the equal dollar allocation to shares of Company X's stock that has a higher beta than Atteric Inc. If everything else remains constant, the required return from the portfolio would