A bicycle wheel, of radius 0.300 m and mass 1.82 kg (concentrated on the rim), is rotating at 4.00 rev/s. After 60.0 s the wheel comes to a stop because of friction. What is the magnitude of the average torque due to frictional forces?

Answer in N?m

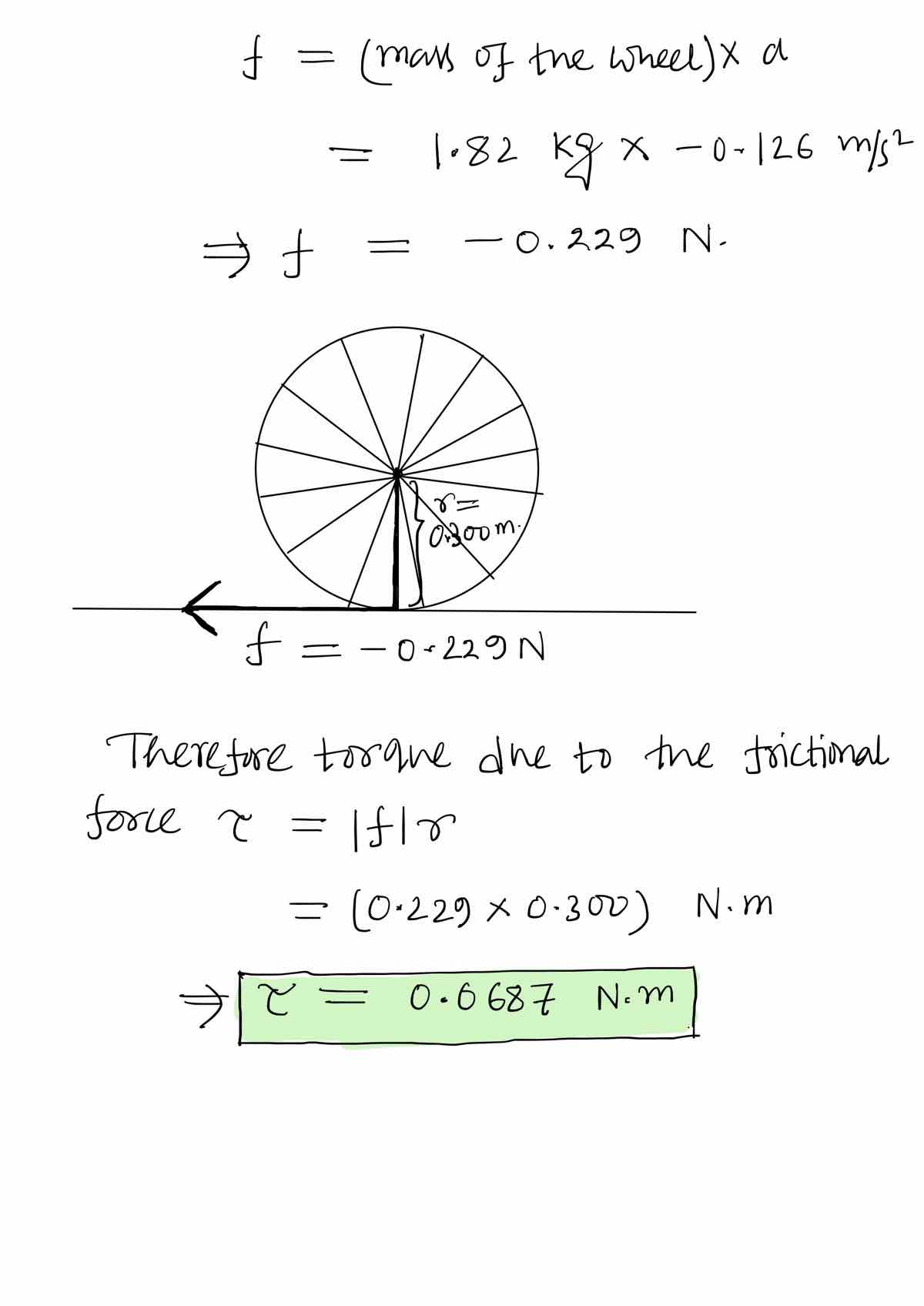A bicycle wheel, of radius 0.300 m and mass 1.82 kg (concentrated on the rim), is rotating at 4.00 rev/s. After 60.0 s the wheel comes to a stop because of friction. What is the magnitude of the average torque clue to frictional forces? -oN-m Solution : - Given that the angular speed of the bicycle W = 4.00 rev/S. = (4:00 x 2TT ) rad /5. LAS, 1 revolution 8 75 ond / 5 . As the radius of the wheel given as r = 0. 300 m. so its linear speed is V = WT - ( 8 1 x 0. 300 ) m/s . V 7- 54 m/s . As the wheel comes to stop after t = 60 0 S . , Let during this time the negative acceleration that acts upon the wheel be a then,by the relation of rectilinear motion, - Vit at Here, Vi = 7- 54 mys (initial velocity of wheel ) and Vf = 0 m /s . (final velocity of wheel) so , 0 = 7. 54 + 60 A = - 7:54 m/jr 60 - 0- 126 m/s2 As only backward frictional force acts on the wheel so we can consider that negative acceleration acts on the wheel due to only folctional force . Therefore the value of backward frictional force on the wheel will bef = ( mass of the wheel ) x d = 1 . 82 Kg x - 0- 126 m/s 2 = - 0. 229 N. 18= 0300 m f = -0-229 N Therefore torque due to the frictional force r = 1518 = (0. 2 29 x 0.300 ) N.M 2 = 0. 6 687 Nom