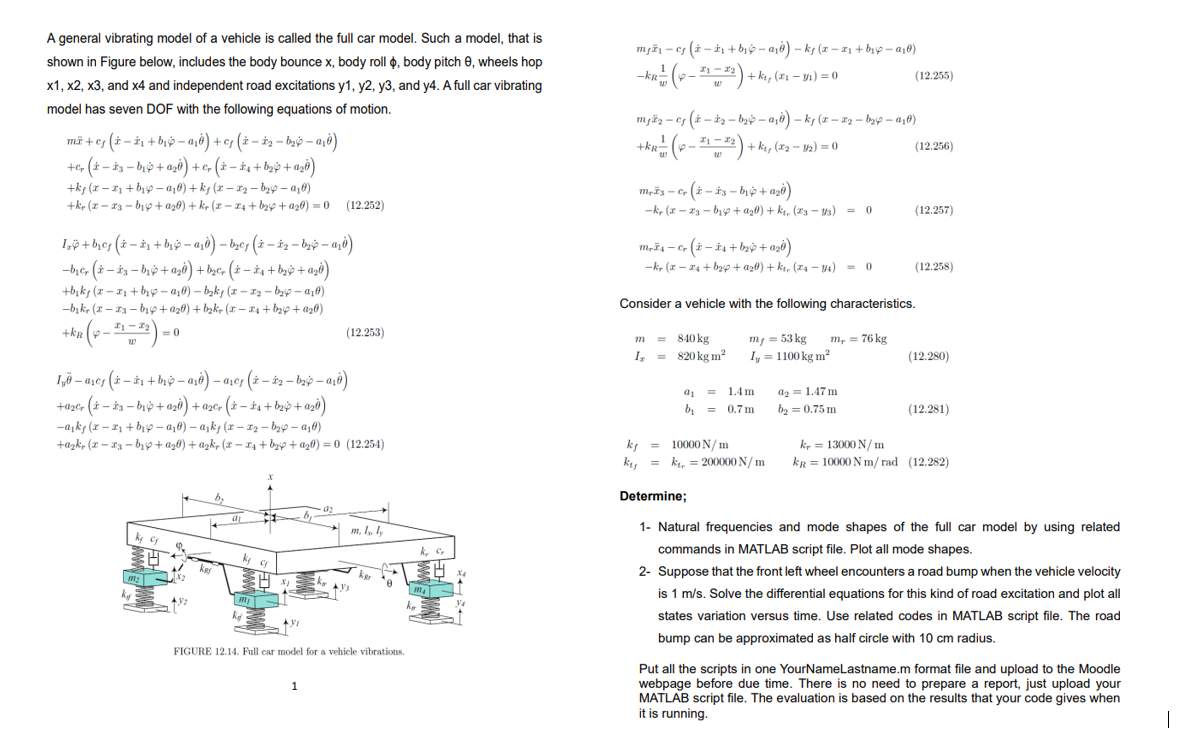
A general vibrating model of a vehicle is called the full car model. Such a model, that is shown in Figure below, includes the body bounce x, body roll , body pitch 8, wheels hop x1, x2, x3, and x4 and independent road excitations y1, y2, y3, and y4. A full car vibrating model has seven DOF with the following equations of motion. m + c (+b-a0) + (-4-0) + ( b + 0) + C ( + +0) G +ky (x+b-a0) + kg (x x b4-010) +kr (2-23-by+ a20) + kr (x x4 + by + a0) = 0 L+ b ( +b 0) byes ( byip 0) -bc ( 3-b+ 0) + bc ( + b + 0) +bk (2- + b - 0) bk (1-1-bp-0) -bkr (xx3-b4+020) + bkr (x x4 +b4+00) +kk (p = - 22 ) = (9- 21- = 0 W ky L-ares (+b-a0) ares (b - 00) +zer (3 + a0) + ac ( ++0) -aky (x-+ 0) ak (x x-0-0) +k, (x-x3-by + a0) + ak, (x x + b+0) = 0 (12.254) kg Cy 10. 432 by K KR a the b. kg cr my (12.252) 431 (12.253) 1 m, I ly FIGURE 12.14. Full car model for a vehicle vibrations. k, Cr m ko m - c (+-0) - k (z-z+b4-10) -kR - (0-1-22) + k, (2-)=0 m - cs (0) k (x x - - 0) +KR (p = 2) + k, (x2 2) = 0 - m - Cr (2-3 -b +00) k (x 23 b4 + 0) + k, (X3 3) = 0 mrs - Cr (2+ bp+ a0) - k (x 24 + b + a20) + k, (x4-4) = 0 - Consider a vehicle with the following characteristics. m = 840 kg mg = 53 kg m = 76 kg Iy = 1100 kg m = 820 kg m I = a = 1.4m b = 0.7m 10000 N/m ke, k =200000N/m (12.255) a=1.47 m b = 0.75m (12.256) (12.257) (12.258) (12.280) (12.281) kg = kp = Determine; 1- Natural frequencies and mode shapes of the full car model by using related commands in MATLAB script file. Plot all mode shapes. 2- Suppose that the front left wheel encounters a road bump when the vehicle velocity is 1 m/s. Solve the differential equations for this kind of road excitation and plot all states variation versus time. Use related codes in MATLAB script file. The road bump can be approximated as half circle with 10 cm radius. k = 13000 N/m = 10000 Nm/rad (12.282) Put all the scripts in one YourNameLastname.m format file and upload to the Moodle webpage before due time. There is no need to prepare a report, just upload your MATLAB script file. The evaluation is based on the results that your code gives when it is running.