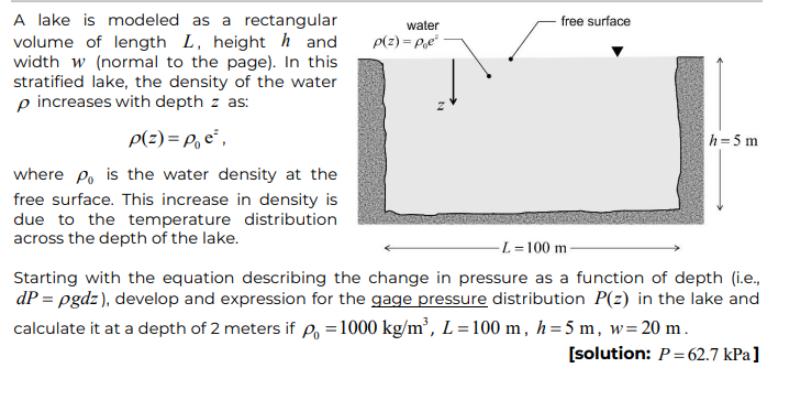
A lake is modeled as a rectangular volume of length L, height hand width w (normal to the page). In this stratified lake, the density of the water p increases with depth z as: p(z) = Pe, where P is the water density at the free surface. This increase in density is due to the temperature distribution across the depth of the lake. water free surface p(z) = Pe -L-100 m- h=5m Starting with the equation describing the change in pressure as a function of depth (i.e., dP = pgdz), develop and expression for the gage pressure distribution P(z) in the lake and calculate it at a depth of 2 meters if p = 1000 kg/m, L=100 m, h=5 m, w=20 m. [solution: P=62.7 kPa]