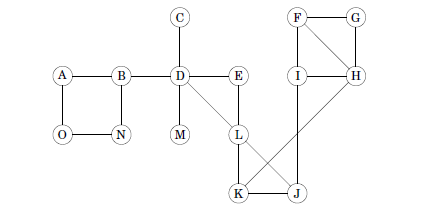
A separating vertex is a vertex whose removal increases the number of connected components in the graph. For example, if the graph were connected (i.e., had just one connected component) then any vertex whose removal disconnects the graph (i.e., leads to two or more connected components) is a separating vertex. (a) To check your understanding of the definition of a separating vertex, find all separating vertices in the graph shown in Problem 3.31 (b) from the textbook. (b) DFS can used to identify separating vertices. To figure out how, first read Section 3.2.4 on the computation of pre(v) and post(v) for each vertex v. Then read the definition of low(u) for a vertex u in Problem 3.31 (g). Modify the functions you wrote in Problem 2 to compute, pre(u), post(u), and low(u) for all vertices u, in linear time (c) Once the values pre(u), post(u), and low(u) for all vertices u are computed, it s pretty easy to use these values to identify separating vertices. Read the characterization of separating vertices in Problem 3.31 (e) and (f). (You dont have to solve these problems; just understand the statements youre being asked to show.) Then modify the explore and DFS functions to return an array of separating vertices. 1 (d) Finally, use your code to output all the separating vertices of the word graph.