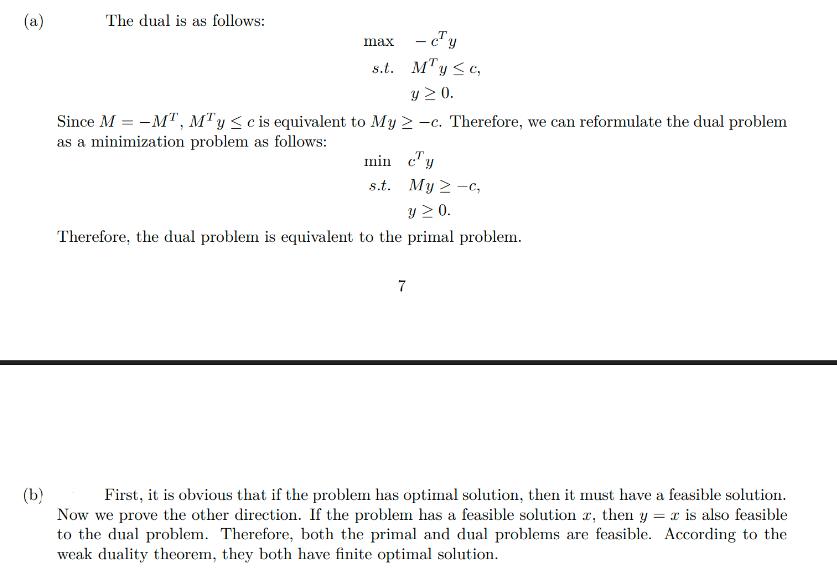
(a) The dual is as follows: max - cy s.t. My c, y 0. Since M = MT, MTy c is equivalent to My 2-c. Therefore, we can reformulate the dual problem as a minimization problem as follows: min s.t. cy My 2-c, y 0. Therefore, the dual problem is equivalent to the primal problem. 7 (b) First, it is obvious that if the problem has optimal solution, then it must have a feasible solution. Now we prove the other direction. If the problem has a feasible solution, then y = r is also feasible to the dual problem. Therefore, both the primal and dual problems are feasible. According to the weak duality theorem, they both have finite optimal solution.