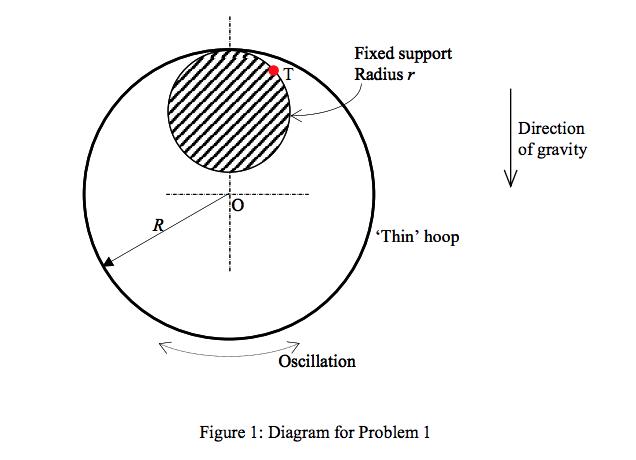
A 'thin' uniform hoop, radius R, is supported on a cylindrical support, radius r, as shown in Figure 1. If deflected slightly in the plane of the page and released, the hoop will oscillate from side to side (assume no slipping). (a) Using a full A4 sheet, draw a large accurate diagram of the system as shown in Figure 1. Superimpose a diagram of the hoop after having rolling to where the point of contact is at T. Draw accurately using compass and straight edge. Lettering may be free-hand. Show all of your geometric constructions neatly on this diagram. (b) For the deflected position, derive an expression for the kinetic energy of the hoop. [10 Marks] (c) For the same position used in (b), derive an expression for the gravitational potential energy of the hoop. [10 Marks] (d) Assuming no damping, apply the principle of conservation of energy to the system to derive the equation of motion. [Energy]=0 dt [7 Marks] (e) Linearise the differential equation obtained in part (d) by assuming small oscillation amplitude. From this linearised differential equation, determine the natural frequency of the small amplitude oscillations. [10 Marks] (f) radius R,) will your mathematical model remain valid if R =R,? If not, what is the new If the hoop were not thin (i.e. it is like a slice of pipe with inside radius R, and outside model? Fixed support Radius r T Direction of gravity R Thin' hoop Oscillation Figure 1: Diagram for Problem 1