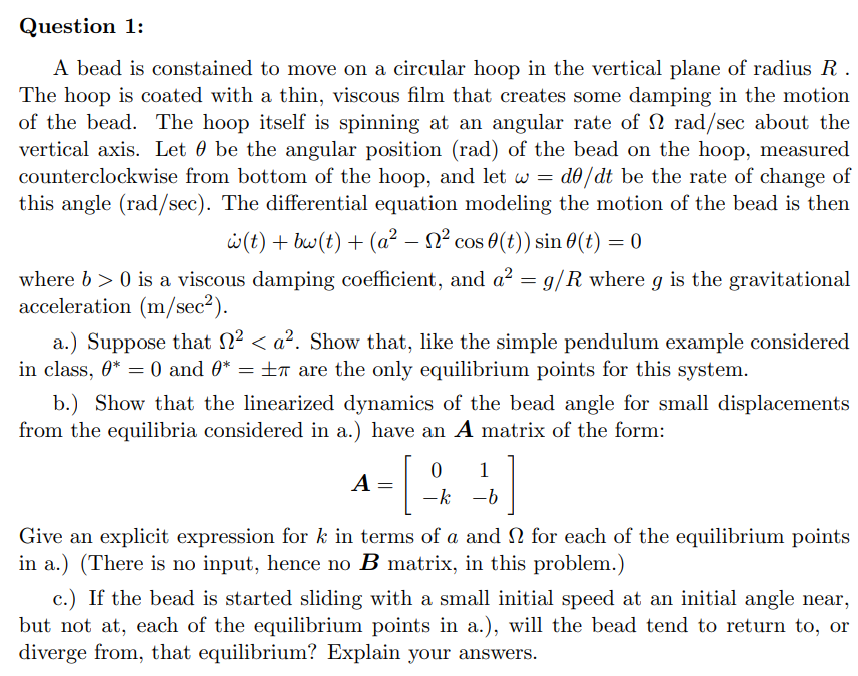
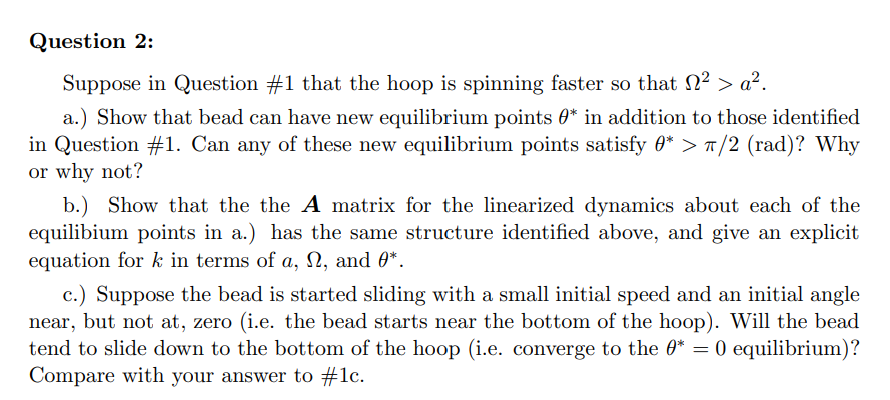
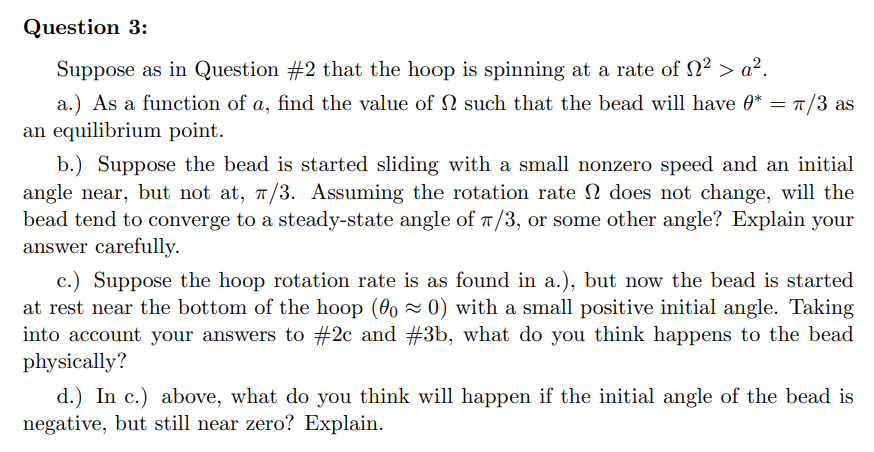
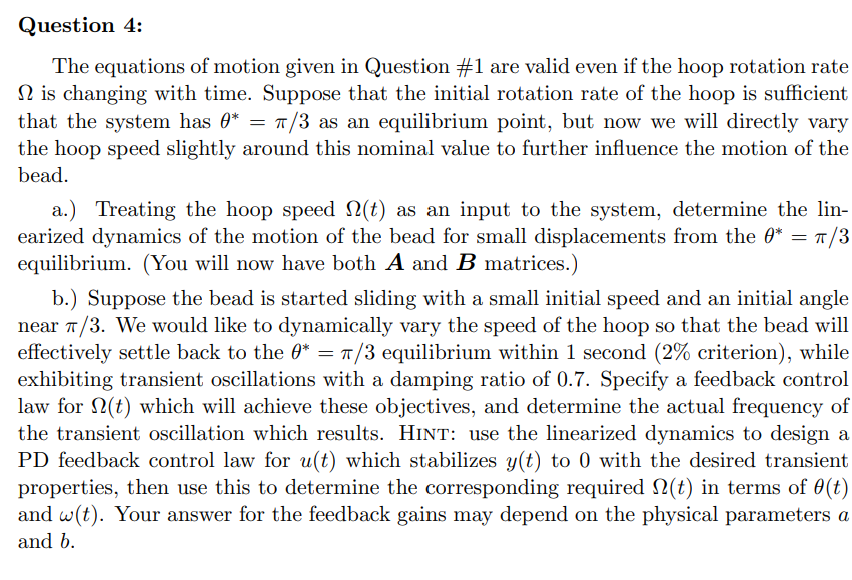
a) To find the equilibrium points of the system, we set the time derivative of the angular velocity, w, to zero: w + bw + = = = (a^2 When w o, the second and third terms on the left-hand side of the equation must cancel each other out: bw + (a^2 Q^2cose) sine - Q^2cose) sine - = There are two possibilities for equilibrium: 1) 0: In this case, we have bw the equation reduces to (a^2 - = 0 = O = Q^2cose) sine = o. Since b > 0 and a^2 > ^2, this equation is satisfied only if sine O, SO 0, which gives = n, where n is an integer. The equilibrium points correspond to o and = , which means ^ = O and 01 TT. 2) bw + (a^2 Q^2cose)sine o: In this pase, if sineo. we can divide the equation by sine and rearrange to get: b/ sine = Q2cose a^2 - The left-hand side is a constant (b/ sine), while the right-hand side depends on 0. Since bo and a^2> ^2, the right-hand side cannot be greater than b/ sine. Therefore, there are no equilibrium points with sine # 0. So, the only equilibrium points for this system are 0^. = 0 and 0^. TT. b) To linearize the dynamics of the bead angle for small displacements from the equilibrium points, we need to linearize the equation around each equilibrium point. Let's consider the linearization around 0^* 0: Linearization around 0^. = = 0: Let 0 0 80, where 80 represents small deviation from the equilibrium value. Similarly, let w w^. + Sw, where Sw , represents a small deviation from the equilibrium angular velocity. Substituting these into the equation of motion and neglecting higher-order terms, we get: = ) (w^. + Sw) + b(w^. + Sw) + (a^2 Q^2cos(8+60)) sin(0^+80) = 0 Expanding the terms and neglecting higher-order small terms, we have: + b = + + Arranging the terms, we obtain: - (a^2 ^2) 0 (a^2 - Q^2)60 Hence, for the linearized dynamics around = 0. the A matrix is: A = [[0, 1]. [-(a^2 - Q^2), -b]] Now let's consider the linearization around 0^-: Linearization around 0^. TT: Using the same procedure as above, we can find that the linearized dynamics for this equilibrium point have the same A matrix: A [[0, 1]. [-(a^2 - 0^2), -b]] Thus, the A matrix is the same for both equilibrium points. c) To determine whether the bead tends to return to or diverge from the equilibrium when started with a small initial speed at an initial angle near but not at the equilibrium points, we can analyze the eigenvalues of the A matrix. For stability, we need the real parts of the eigenvalues to be negative. If of the any eigenvalues has a positive real part, the system will be unstable. Question 1: A bead is constained to move on a circular hoop in the vertical plane of radius R The hoop is coated with a thin, viscous film that creates some damping in the motion of the bead. The hoop itself is spinning at an angular rate of rad/sec about the vertical axis. Let be the angular position (rad) of the bead on the hoop, measured counterclockwise from bottom of the hoop, and let w = do/dt be the rate of change of this angle (rad/sec). The differential equation modeling the motion of the bead is then w(t) + bw (t) + (a-N cos 0 (t)) sin 0(t) = 0 where b> 0 is a viscous damping coefficient, and a = g/R where g is the gravitational acceleration (m/sec). a.) Suppose that N < a. Show that, like the simple pendulum example considered in class, 0* = 0 and 0* = are the only equilibrium points for this system. b.) Show that the linearized dynamics of the bead angle for small displacements from the equilibria considered in a.) have an A matrix of the form: 0 1 -k-b A [ = Give an explicit expression for k in terms of a and 2 for each of the equilibrium points in a.) (There is no input, hence no B matrix, in this problem.) c.) If the bead is started sliding with a small initial speed at an initial angle near, but not at, each of the equilibrium points in a.), will the bead tend to return to, or diverge from, that equilibrium? Explain your answers. Question 2: Suppose in Question #1 that the hoop is spinning faster so that N > a. a.) Show that bead can have new equilibrium points 0* in addition to those identified in Question #1. Can any of these new equilibrium points satisfy 0* > /2 (rad)? Why or why not? b.) Show that the the A matrix for the linearized dynamics about each of the equilibium points in a.) has the same structure identified above, and give an explicit equation for k in terms of a, , and 0*. c.) Suppose the bead is started sliding with a small initial speed and an initial angle near, but not at, zero (i.e. the bead starts near the bottom of the hoop). Will the bead tend to slide down to the bottom of the hoop (i.e. converge to the 0* = 0 equilibrium)? Compare with your answer to #1c. Question 3: Suppose as in Question #2 that the hoop is spinning at a rate of > a. a.) As a function of a, find the value of such that the bead will have 0* = /3 as an equilibrium point. b.) Suppose the bead is started sliding with a small nonzero speed and an initial angle near, but not at, 7/3. Assuming the rotation rate does not change, will the bead tend to converge to a steady-state angle of T/3, or some other angle? Explain your answer carefully. c.) Suppose the hoop rotation rate is as found in a.), but now the bead is started at rest near the bottom of the hoop (000) with a small positive initial angle. Taking into account your answers to #2c and #3b, what do you think happens to the bead physically? d.) In c.) above, what do you think will happen if the initial angle of the bead is negative, but still near zero? Explain. Question 4: The equations of motion given in Question #1 are valid even if the hoop rotation rate is changing with time. Suppose that the initial rotation rate of the hoop is sufficient that the system has 0* = /3 as an equilibrium point, but now we will directly vary the hoop speed slightly around this nominal value to further influence the motion of the bead. a.) Treating the hoop speed (t) as an input to the system, determine the lin- earized dynamics of the motion of the bead for small displacements from the 0* = /3 equilibrium. (You will now have both A and B matrices.) b.) Suppose the bead is started sliding with a small initial speed and an initial angle near T/3. We would like to dynamically vary the speed of the hoop so that the bead will effectively settle back to the 0* = /3 equilibrium within 1 second (2% criterion), while exhibiting transient oscillations with a damping ratio of 0.7. Specify a feedback control law for (t) which will achieve these objectives, and determine the actual frequency of the transient oscillation which results. HINT: use the linearized dynamics to design a PD feedback control law for u(t) which stabilizes y(t) to 0 with the desired transient properties, then use this to determine the corresponding required (t) in terms of (t) and w(t). Your answer for the feedback gains may depend on the physical parameters a and b.