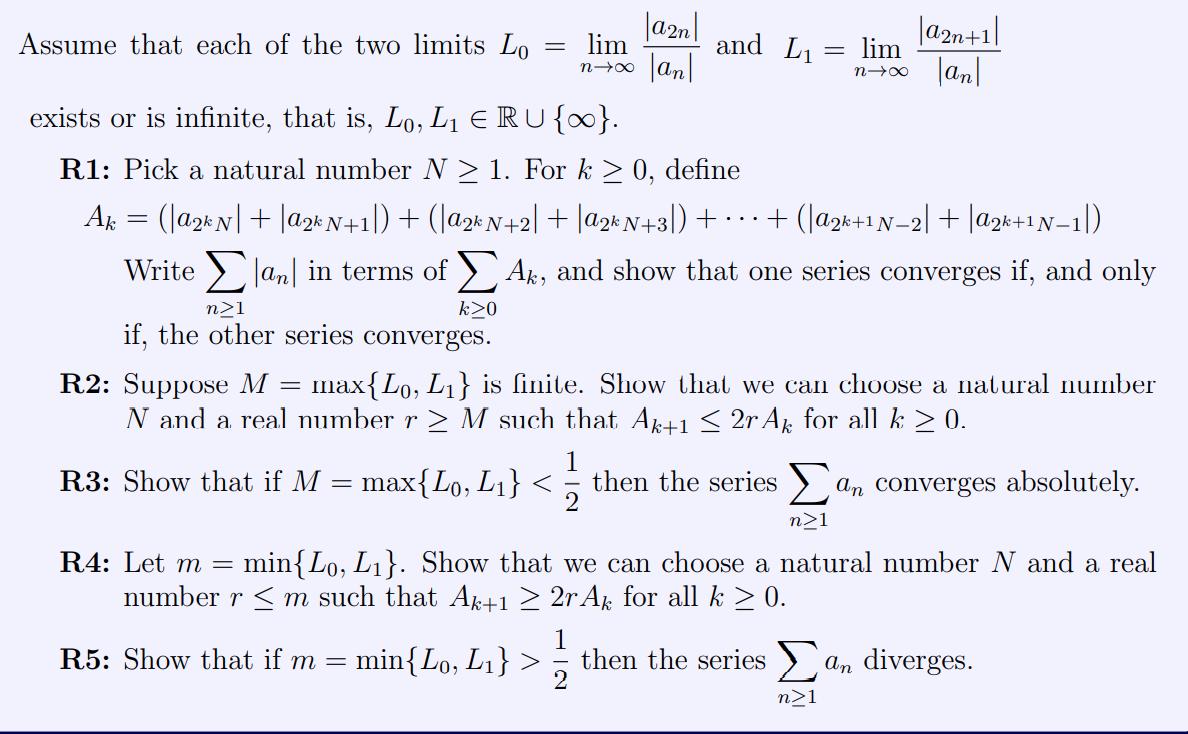
Assume that each of the two limits Lo = lim n n>1 if, the other series converges. |a2n| |an| and L lim n |a2n+1| |an| exists or is infinite, that is, Lo, L RU{}. R1: Pick a natural number N 1. For k 0, define Ak = = (|akN| + |akN+1]) + (|akN+2] + [a2kN+3|) + + (|a2k+1N_2| + |a2k+N1|) Write an in terms of Ak, and show that one series converges if, and only k>0 R2: Suppose M = max{Lo, L} is finite. Show that we can choose a natural number N and a real number r M such that Ak+1 2r Ak for all k 0. 1 R3: Show that if M max{Lo, L} < then the series an converges absolutely. n>1 R4: Let m = min{Lo, L}. Show that we can choose a natural number N and a real number r m such that Ak+1 2r Ak for all k 0. 1 R5: Show that if m= min{Lo, L} > then the series 2 n>1 an diverges.