About Lagrange Interpolation:

Please code in C or C++

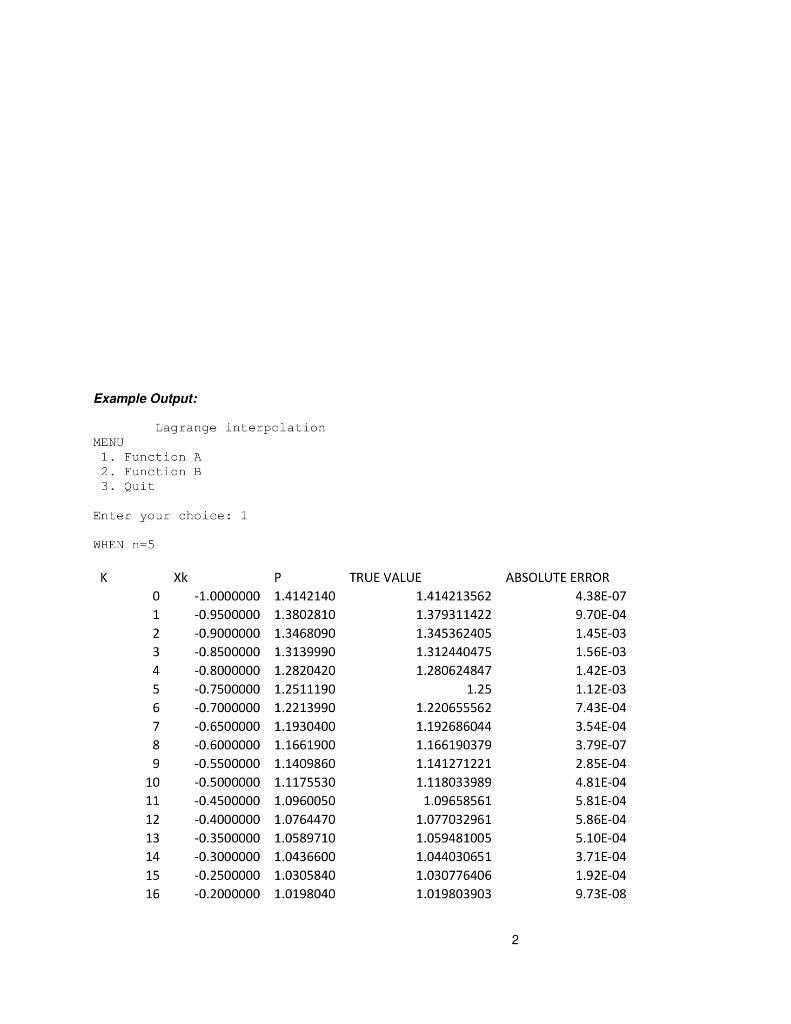
Example Output: Lagrange interpolation MENU 1. Function A 2. Function B 3. Quit Enter your choice: 1 WHEN n=5 K Xk P TRUE VALUE ABSOLUTE ERROR 0 -1.0000000 1.4142140 1.414213562 4.38E-07 1 -0.9500000 1.3802810 1.379311422 9.70E-04 2 -0.9000000 1.3468090 1.345362405 1.45E-03 3 -0.8500000 1.3139990 1.312440475 1.56E-03 4 -0.8000000 1.2820420 1.280624847 1.42E-03 5 -0.7500000 1.2511190 1.25 1.12E-03 6 -0.7000000 1.2213990 1.220655562 7.43E-04 7 -0.6500000 1.1930400 1.192686044 3.54E-04 8 -0.6000000 1.1661900 1.166190379 3.79E-07 9 -0.5500000 1.1409860 1.141271221 2.85E-04 10 -0.5000000 1.1175530 1.118033989 4.81E-04 11 -0.4500000 1.0960050 1.09658561 5.81E-04 12 -0.4000000 1.0764470 1.077032961 5.86E-04 13 -0.3500000 1.0589710 1.059481005 5.10E-04 14 -0.3000000 1.0436600 1.044030651 3.71E-04 15 -0.2500000 1.0305840 1.030776406 1.92E-04 16 -0.2000000 1.0198040 1.019803903 9.73E-08

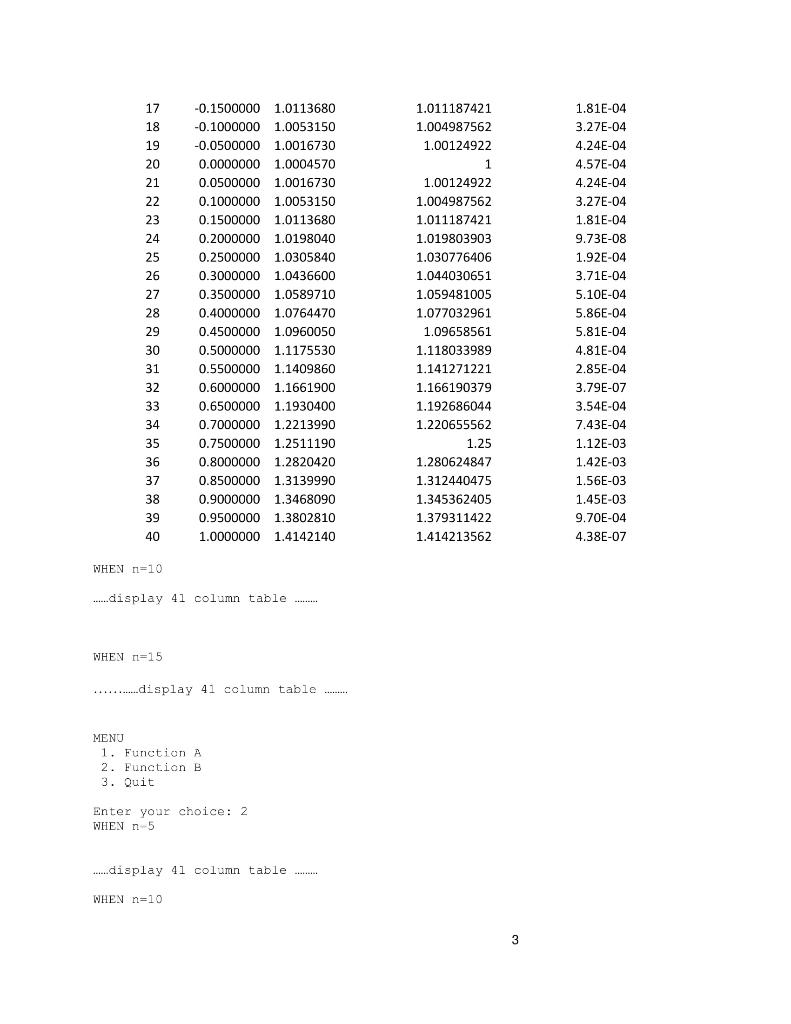
17 -0.1500000 1.0113680 1.011187421 1.81E-04 18 -0.1000000 1.0053150 1.004987562 3.27E-04 19 -0.0500000 1.0016730 1.00124922 4.24E-04 20 0.0000000 1.0004570 1 4.57E-04 21 0.0500000 1.0016730 1.00124922 4.24E-04 22 0.1000000 1.0053150 1.004987562 3.27E-04 23 0.1500000 1.0113680 1.011187421 1.81E-04 24 0.2000000 1.0198040 1.019803903 9.73E-08 25 0.2500000 1.0305840 1.030776406 1.92E-04 26 0.3000000 1.0436600 1.044030651 3.71E-04 27 0.3500000 1.0589710 1.059481005 5.10E-04 28 0.4000000 1.0764470 1.077032961 5.86E-04 29 0.4500000 1.0960050 1.09658561 5.81E-04 30 0.5000000 1.1175530 1.118033989 4.81E-04 31 0.5500000 1.1409860 1.141271221 2.85E-04 32 0.6000000 1.1661900 1.166190379 3.79E-07 33 0.6500000 1.1930400 1.192686044 3.54E-04 34 0.7000000 1.2213990 1.220655562 7.43E-04 35 0.7500000 1.2511190 1.25 1.12E-03 36 0.8000000 1.2820420 1.280624847 1.42E-03 37 0.8500000 1.3139990 1.312440475 1.56E-03 38 0.9000000 1.3468090 1.345362405 1.45E-03 39 0.9500000 1.3802810 1.379311422 9.70E-04 40 1.0000000 1.4142140 1.414213562 4.38E-07 WHEN n=10 display 41 column table WHEN n=15 display 41 column table MENU 1. Function A 2. Function B 3. Quit Enter your choice: 2 WHEN n=5 display 41 column table WHEN n=10 display 41 column table WHEN n=15 display 41 column table MENU 1. Function A 2. Function B 3. Quit Enter your choice: 3 Exit

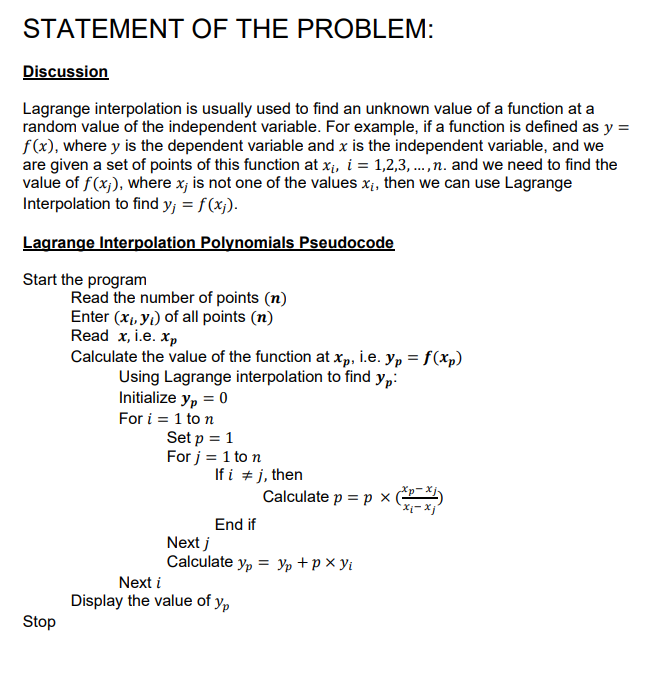
STATEMENT OF THE PROBLEM: Discussion Lagrange interpolation is usually used to find an unknown value of a function at a random value of the independent variable. For example, if a function is defined as y = f(x), where y is the dependent variable and x is the independent variable, and we are given a set of points of this function at Xi, i = 1,2,3,..., n. and we need to find the value of f(x;), where x; is not one of the values xi, then we can use Lagrange Interpolation to find y; = f(x;). Lagrange Interpolation Polynomials Pseudocode Start the program Read the number of points (n) Enter (x,y) of all points (n) Read x, i.e. Xp Calculate the value of the function at Xp, i.e. Yp = f(xp) Using Lagrange interpolation to find yp: Initialize yp = 0 For i = 1 ton Set p = 1 For j = 1 to n If i #j, then Calculate p = p xp-x) *1-*/ End if Next j Calculate yp = Yp + p X Yi Next i Display the value of yp Stop Problem Description: Use Lagrange interpolation to interpolate the following functions: (a) f(x) = V1 + x2 (b) f(x) = 1 1+25x2 using a set of n+1 regularly spaced nodes computed by the following equation: 2(k - 1) Xk = -1+ -, k = 1,2,3,......, n +1 n Test your generated polynomial with different orders, n= 5, 10, 20 and compute the interpolation polynomial Pn(x) at 41 regularly spaced points. For each value of xk the Lagrange polynomial approximation is output together with the exact /true value from the math library, also output the absolute error. Example Output: Lagrange interpolation MENU 1. Function A 2. Function 3. Quit Enter your choice: 1 WHEN 5 K Xk 0 1 2 3 4 5 6 7 P -1.0000000 1.4142140 -0.9500000 1.3802810 -0.9000000 1.3468090 -0.8500000 1.3139990 -0.8000000 1.2820420 -0.7500000 1.2511190 -0.7000000 1.2213990 -0.6500000 1.1930400 -0.6000000 1.1661900 -0.5500000 1.1409860 -0.5000000 1.1175530 -0.4500000 1.0960050 -0.4000000 1.0764470 -0.3500000 1.0589710 -0.3000000 1.0436600 .2500000 1.0305840 -0.2000000 1.0198040 TRUE VALUE 1.414213562 1.379311422 1.345362405 1.312440475 1.280624847 1.25 1.220655562 1.192686044 1.166190379 1.141271221 1.118033989 1.09658561 1.077032961 1.059481005 1.044030651 1.030776406 1.019803903 ABSOLUTE ERROR 4.38E-07 9.70E-04 1.45E-03 1.56E-03 1.42E-03 1.12E-03 7.43E-04 3.54E-04 3.79E-07 2.85E-04 4.81E-04 5.81E-04 5.86E-04 5.10E-04 3.71E-04 1.92E-04 9.73E-08 8 9 10 11 12 13 14 15 16 2 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 -0.1500000 1.0113680 -0.1000000 1.0053150 -0.0500000 1.0016730 0.0000000 1.0004570 0.0500000 1.0016730 0.1000000 1.0053150 0.1500000 1.0113680 0.2000000 1.0198040 0.2500000 1.0305840 0.3000000 1.0436600 0.3500000 1.0589710 0.4000000 1.0764470 0.4500000 1.0960050 0.5000000 1.1175530 0.5500000 1.1409860 0.6000000 1.1661900 0.6500000 1.1930400 0.7000000 1.2213990 0.7500000 1.2511190 0.8000000 1.2820420 0.8500000 1.3139990 0.9000000 1.3468090 0.9500000 1.3802810 1.0000000 1.4142140 1.011187421 1.004987562 1.00124922 1 1.00124922 1.004987562 1.011187421 1.019803903 1.030776406 1.044030651 1.059481005 1.077032961 1.09658561 1.118033989 1.141271221 1.166190379 1.192686044 1.220655562 1.25 1.280624847 1.312440475 1.345362405 1.379311422 1.414213562 1.81E-04 3.27E-04 4.24E-04 4.57E-04 4.24E-04 3.27E-04 1.81E-04 9.73E-08 1.92E-04 3.71E-04 5.10E-04 5.86E-04 5.81E-04 4.81E-04 2.85E-04 3.79E-07 3.54E-04 7.43E-04 1.12E-03 1.42E-03 1.56E-03 1.45E-03 9.70E-04 4.38E-07 WHEN n-10 .....display 41 column table....... WHEN n=15 ..........display 41 column table ........ MENU 1. Function A 2. Function B 3. Quit Enter your choice: 2 WHEN n 5 ......display 41 column table WHEN n=10 3 ...display 41 column table ........ WHEN 1=15 ......display 41 column table MENO 1. Function A 2. Function B 3. Quit Enter your choice: 3 Exit 4 STATEMENT OF THE PROBLEM: Discussion Lagrange interpolation is usually used to find an unknown value of a function at a random value of the independent variable. For example, if a function is defined as y = f(x), where y is the dependent variable and x is the independent variable, and we are given a set of points of this function at Xi, i = 1,2,3,..., n. and we need to find the value of f(x;), where x; is not one of the values xi, then we can use Lagrange Interpolation to find y; = f(x;). Lagrange Interpolation Polynomials Pseudocode Start the program Read the number of points (n) Enter (x,y) of all points (n) Read x, i.e. Xp Calculate the value of the function at Xp, i.e. Yp = f(xp) Using Lagrange interpolation to find yp: Initialize yp = 0 For i = 1 ton Set p = 1 For j = 1 to n If i #j, then Calculate p = p xp-x) *1-*/ End if Next j Calculate yp = Yp + p X Yi Next i Display the value of yp Stop Problem Description: Use Lagrange interpolation to interpolate the following functions: (a) f(x) = V1 + x2 (b) f(x) = 1 1+25x2 using a set of n+1 regularly spaced nodes computed by the following equation: 2(k - 1) Xk = -1+ -, k = 1,2,3,......, n +1 n Test your generated polynomial with different orders, n= 5, 10, 20 and compute the interpolation polynomial Pn(x) at 41 regularly spaced points. For each value of xk the Lagrange polynomial approximation is output together with the exact /true value from the math library, also output the absolute error. Example Output: Lagrange interpolation MENU 1. Function A 2. Function 3. Quit Enter your choice: 1 WHEN 5 K Xk 0 1 2 3 4 5 6 7 P -1.0000000 1.4142140 -0.9500000 1.3802810 -0.9000000 1.3468090 -0.8500000 1.3139990 -0.8000000 1.2820420 -0.7500000 1.2511190 -0.7000000 1.2213990 -0.6500000 1.1930400 -0.6000000 1.1661900 -0.5500000 1.1409860 -0.5000000 1.1175530 -0.4500000 1.0960050 -0.4000000 1.0764470 -0.3500000 1.0589710 -0.3000000 1.0436600 .2500000 1.0305840 -0.2000000 1.0198040 TRUE VALUE 1.414213562 1.379311422 1.345362405 1.312440475 1.280624847 1.25 1.220655562 1.192686044 1.166190379 1.141271221 1.118033989 1.09658561 1.077032961 1.059481005 1.044030651 1.030776406 1.019803903 ABSOLUTE ERROR 4.38E-07 9.70E-04 1.45E-03 1.56E-03 1.42E-03 1.12E-03 7.43E-04 3.54E-04 3.79E-07 2.85E-04 4.81E-04 5.81E-04 5.86E-04 5.10E-04 3.71E-04 1.92E-04 9.73E-08 8 9 10 11 12 13 14 15 16 2 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 -0.1500000 1.0113680 -0.1000000 1.0053150 -0.0500000 1.0016730 0.0000000 1.0004570 0.0500000 1.0016730 0.1000000 1.0053150 0.1500000 1.0113680 0.2000000 1.0198040 0.2500000 1.0305840 0.3000000 1.0436600 0.3500000 1.0589710 0.4000000 1.0764470 0.4500000 1.0960050 0.5000000 1.1175530 0.5500000 1.1409860 0.6000000 1.1661900 0.6500000 1.1930400 0.7000000 1.2213990 0.7500000 1.2511190 0.8000000 1.2820420 0.8500000 1.3139990 0.9000000 1.3468090 0.9500000 1.3802810 1.0000000 1.4142140 1.011187421 1.004987562 1.00124922 1 1.00124922 1.004987562 1.011187421 1.019803903 1.030776406 1.044030651 1.059481005 1.077032961 1.09658561 1.118033989 1.141271221 1.166190379 1.192686044 1.220655562 1.25 1.280624847 1.312440475 1.345362405 1.379311422 1.414213562 1.81E-04 3.27E-04 4.24E-04 4.57E-04 4.24E-04 3.27E-04 1.81E-04 9.73E-08 1.92E-04 3.71E-04 5.10E-04 5.86E-04 5.81E-04 4.81E-04 2.85E-04 3.79E-07 3.54E-04 7.43E-04 1.12E-03 1.42E-03 1.56E-03 1.45E-03 9.70E-04 4.38E-07 WHEN n-10 .....display 41 column table....... WHEN n=15 ..........display 41 column table ........ MENU 1. Function A 2. Function B 3. Quit Enter your choice: 2 WHEN n 5 ......display 41 column table WHEN n=10 3 ...display 41 column table ........ WHEN 1=15 ......display 41 column table MENO 1. Function A 2. Function B 3. Quit Enter your choice: 3 Exit 4