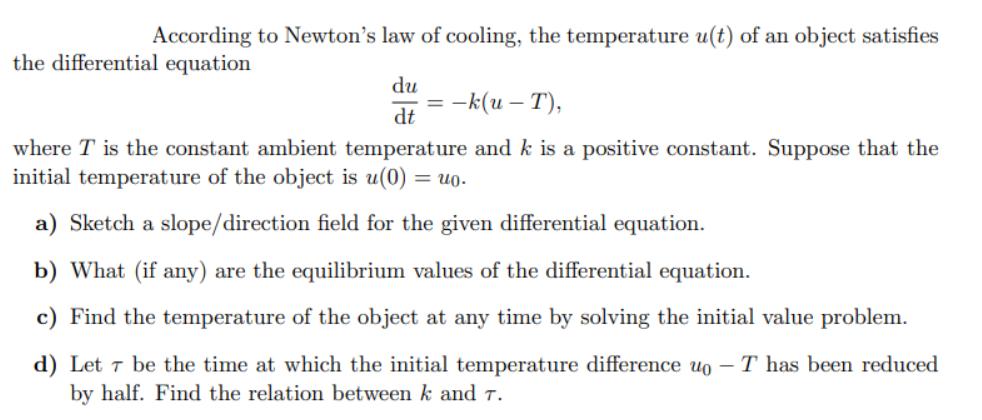
According to Newton's law of cooling, the temperature u(t) of an object satisfies the differential equation du dt = -k(u-T), where T is the constant ambient temperature and k is a positive constant. Suppose that the initial temperature of the object is u(0) = uo. a) Sketch a slope/direction field for the given differential equation. b) What (if any) are the equilibrium values of the differential equation. c) Find the temperature of the object at any time by solving the initial value problem. d) Let 7 be the time at which the initial temperature difference uo- T has been reduced by half. Find the relation between k and 7.