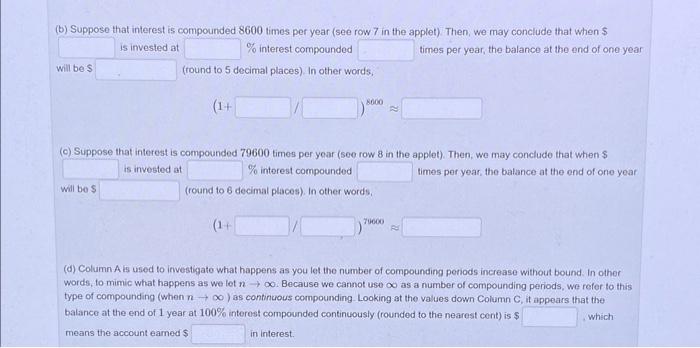
ADTF Distributors is opening a corporate office building in Kansas, near the campus of CLU. They contact the business college at CLU to solicit applications from students for consideration for several openings in the Finance division at the new site. Applicants invited to the first round of interviews were asked to use the block of cells A1-C11 in the above spreadsheet applet. These cells are programmed to display data for a scenario where $1 is invested at 100% interest compounded 7 times per year. Column A gives possible values for the number of compounding periods and Column C gives the balance at the end of 1 year. Note: There are formulas entered in Columns B and C of row 3. If you delete the data in these cells, you can refresh the applet (or reload the page) to restore them. If you grab the lower right corner of a cell with a formula, you can drag the formula to fill down the column. For example, you could do this with cell B3. See how well you would perform at the first round of interviews by completing the exercises below. INTERVIEW - ROUND ONE (a) Let n stand for the number of compounding periods. Then, using the compound interest formula, the future value when $1 is invested at 100% interest for 1 year will be J. (1+ AC[ This simplifies to (b) Suppose that interest is compounded 8600 times per year (see row 7 in the applet). Then, we may conclude that when S is invested at % interest compounded times per year, the balance at the end of one year (round to 5 decimal places). In other words, will be $ (1+ will be $ (c) Suppose that interest is compounded 79600 times per year (see row 8 in the applet). Then, we may conclude that when $ % interest compounded is invested at times per year, the balance at the end of one year. (round to 6 decimal places). In other words, 8000 (1+ 79000 RU (d) Column A is used to investigate what happens as you let the number of compounding periods increase without bound. In other i words, to mimic what happens as we let noo. Because we cannot use oo as a number of compounding periods, we refer to this type of compounding (when noo) as continuous compounding. Looking at the values down Column C, it appears that the balance at the end of 1 year at 100% interest compounded continuously (rounded to the nearest cent) is $ which means the account earned $ in interest. (e) Correct to 5 decimal places, it appears that as n 00, Did you conclude that the limiting value of (1+1/n)" as n oo is equal to e (the natural base)? Then, congratulations, you are moving on to the second round of interviews. INTERVIEW - ROUND TWO n (+1)^{ Applicants invited to the second round of interviews were asked to use the block of cells A16-C24 in the above spreadsheet applet. These cells are programmed to display data for a scenario where $1 is invested at 19% interest compounded in times per year. Column A gives possible values for the number of compounding periods and Column C gives the balance at the end of 1 year. Note: There are formulas entered in Columns B and C of row 17. If you delete the data in these cells, you can refresh the applet (or reload the page) to restore them. If you grab the lower right corner of a cell with a formula, you can drag the formula to fill down the column. For example, you could do this with cell B18 into B19, (f) Let n stand for the number of compounding periods. Then, using the compound interest formula, the future value when $1 is invested at 19% interest for 1 year will be -(1+[ ([ This simplifies to 0.19 n (g) Suppose that interest is compounded 8600 times per year (see row 20 in the applet). Then, we may conclude that when $ is invested at % interest compounded times per year, the balance at the end of one year (round to 5 decimal places). In other words, will be $ will be $ (h) Suppose that interest is compounded 79600 times per year (see row 21 in the applet). Then, we may conclude that when $ % interest compounded is invested at times per year, the balance at the end of one year (round to 6 decimal places). In other words, (1+ () Correct to 5 decimal places, it appears that as n -> 00, 8600 P 1 Z 79000 (1) Column A is used to investigate what happens as you let the number of compounding periods increase without bound. In other. words, to mimic what happens as we let noo. Because we cannot use oo as a number of compounding periods, we refer to this type of compounding (when 12.00) as continuous compounding. Looking at the values down Column C. it appears that the balance at the end of 1 year at 19% interest compounded continuously (rounded to the nearest cont) is $ means the account earned $ which in interest B I Did you conclude that the limiting value of (1 +0.19/n)" as noo is equal to 192 Then, congratulations, you have passed the second round of interviews. Watch the mail for an offer letter from ADTF Distributors.