ALL ABOUT STATS - NO EXPLANATION NEEDED
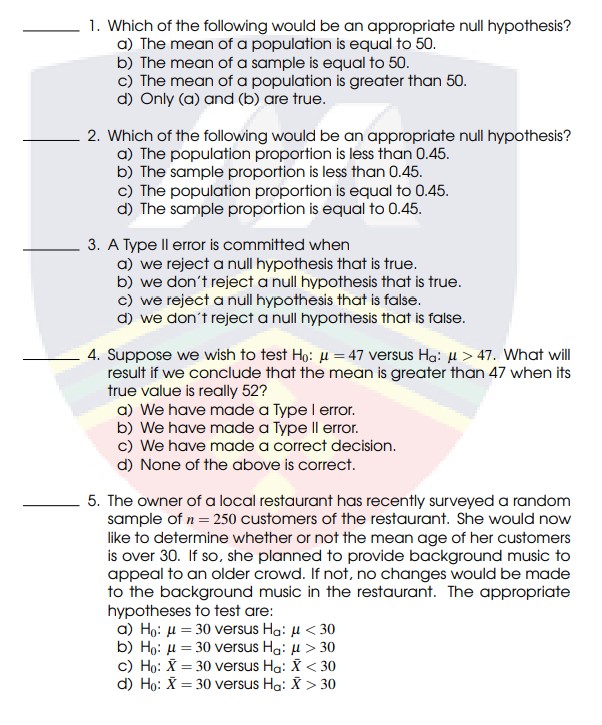
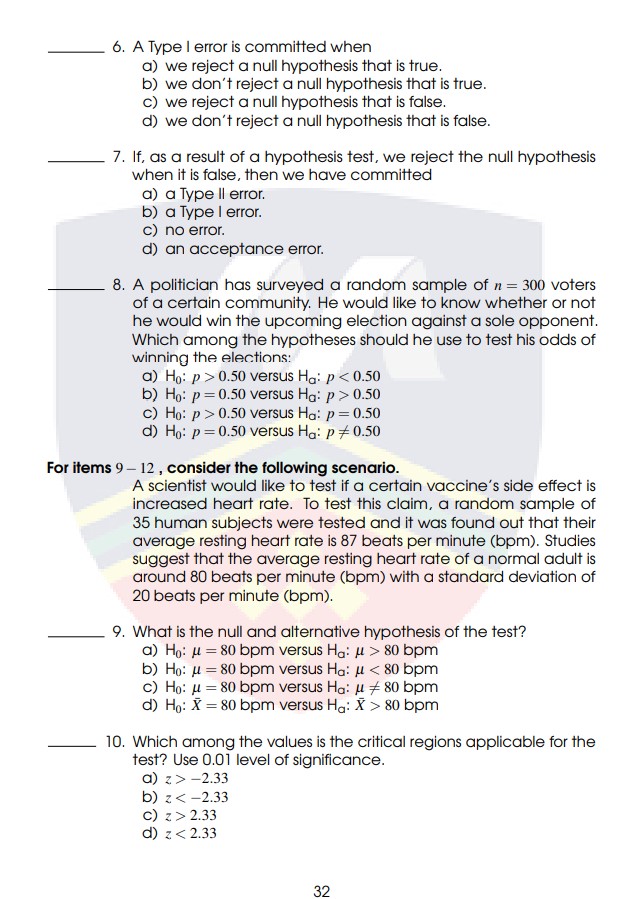
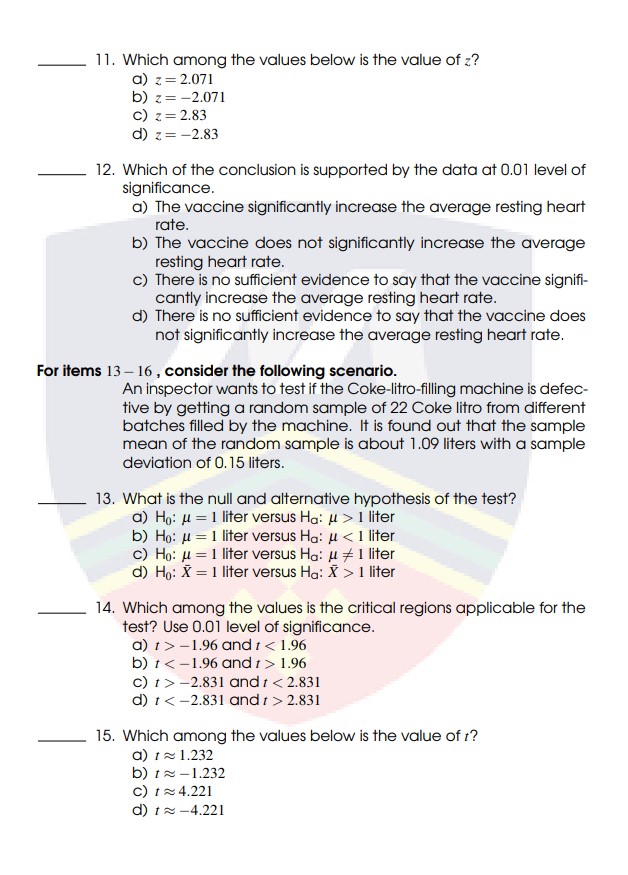
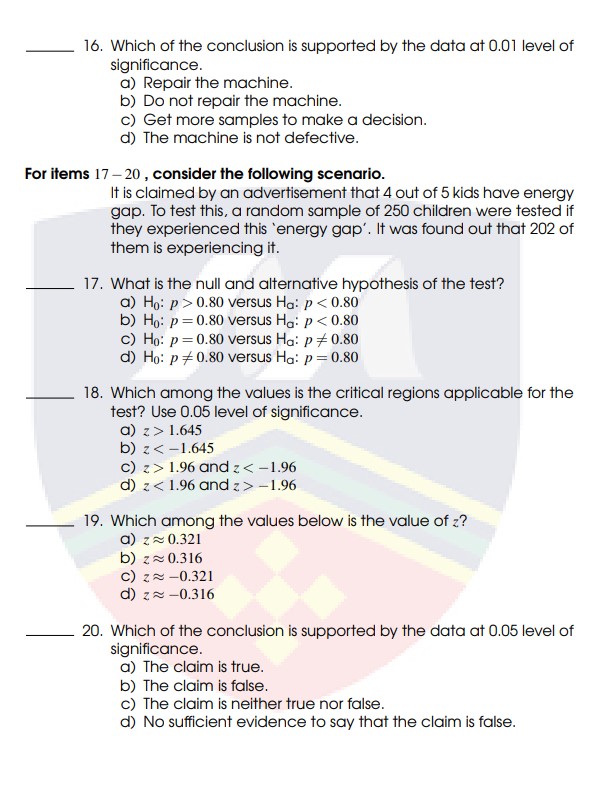
1. Which of the following would be an appropriate null hypothesis? a) The mean of a population is equal to 50. b) The mean of a sample is equal to 50. c) The mean of a population is greater than 50. d) Only (a) and (b) are true. 2. Which of the following would be an appropriate null hypothesis? a) The population proportion is less than 0.45. b) The sample proportion is less than 0.45. c) The population proportion is equal to 0.45. d) The sample proportion is equal to 0.45. 3. A Type ll error is committed when a) we reject a null hypothesis that is true. b) we don't reject a null hypothesis that is true. c) we reject a null hypothesis that is false. d) we don't reject a null hypothesis that is false. 4. Suppose we wish to test Ho: u = 47 versus Ha: u > 47. What will result if we conclude that the mean is greater than 47 when its true value is really 52? a) We have made a Type | error. b) We have made a Type II error. c) We have made a correct decision. d) None of the above is correct. 5. The owner of a local restaurant has recently surveyed a random sample of n = 250 customers of the restaurant. She would now like to determine whether or not the mean age of her customers is over 30. If so, she planned to provide background music to appeal to an older crowd. If not, no changes would be made to the background music in the restaurant. The appropriate hypotheses to test are: a) Ho: H = 30 versus Ha: H 30 C) Ho: X = 30 versus Ha: X 306. A Type I error is committed when a) we reject a null hypothesis that is true. b) we don't reject a null hypothesis that is true. c) we reject a null hypothesis that is false. d) we don't reject a null hypothesis that is false. 7. If, as a result of a hypothesis test, we reject the null hypothesis when it is false, then we have committed a) a Type ll error. b) a Type I error. c) no error. d) an acceptance error. 8. A politician has surveyed a random sample of n = 300 voters of a certain community. He would like to know whether or not he would win the upcoming election against a sole opponent. Which among the hypotheses should he use to test his odds of winning the elections: a) Ho: p > 0.50 versus Ha: p 0.50 c) Ho: p > 0.50 versus Ha: p = 0.50 d) Ho: p = 0.50 versus Ha: p # 0.50 For items 9- 12 , consider the following scenario. A scientist would like to test if a certain vaccine's side effect is increased heart rate. To test this claim, a random sample of 35 human subjects were tested and it was found out that their average resting heart rate is 87 beats per minute (bpm). Studies suggest that the average resting heart rate of a normal adult is around 80 beats per minute (bpm) with a standard deviation of 20 beats per minute (bpm). 9. What is the null and alternative hypothesis of the test? a) Ho: u = 80 bpm versus Ha: # > 80 bpm b) Ho: u = 80 bpm versus Ha: / 80 bpm 10. Which among the values is the critical regions applicable for the test? Use 0.01 level of significance. a) z> -2.33 b) z 2.33 d) z 1 liter b) Ho: u = 1 liter versus Ha: u 1 liter 14. Which among the values is the critical regions applicable for the test? Use 0.01 level of significance. a) 1> -1.96 and r 1.96 C) 1 > -2.831 and / 2.831 15. Which among the values below is the value of ? a) 1=1.232 b) 1 2 -1.232 C) 1 24.221 d) 12-4.22116. Which of the conclusion is supported by the data at 0.01 level of significance. a) Repair the machine. b) Do not repair the machine. c) Get more samples to make a decision. d) The machine is not defective. For items 17-20, consider the following scenario. It is claimed by an advertisement that 4 out of 5 kids have energy gap. To test this, a random sample of 250 children were tested if they experienced this 'energy gap'. It was found out that 202 of them is experiencing it. 17. What is the null and alternative hypothesis of the test? a) Ho: p > 0.80 versus Ha: p 1.645 b) z 1.96 and z -1.96 19. Which among the values below is the value of z? a) z= 0.321 b) z= 0.316 C) 28-0.321 d) zz-0.316 20. Which of the conclusion is supported by the data at 0.05 level of significance. a) The claim is true. b) The claim is false. c) The claim is neither true nor false. d) No sufficient evidence to say that the claim is false