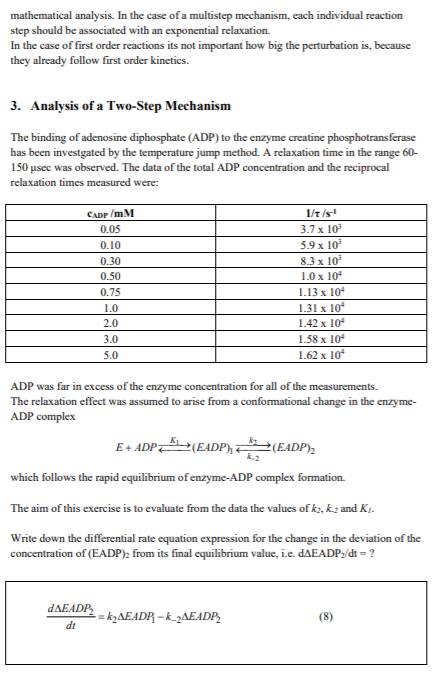
There are many reactions which proceed on a sub-second timescale, even down to femtoseconds. For such fast reactions it is not possible to simply mix reactants by hand and measure the amount of product formed as a function of time. Specialized fast reaction techniques are required. There are two major classes of fast reaction techniques: 1) fast flow techniques (e.g. continuous-flow, stopped-flow) 2) perturbation techniques In flow techniques two solutions (or gases) are rapidly mixed by using hydraulic air pressure. The reaction following mixing is then often observed spectroscopically or conductimetrically. In the continuous-flow method the solutions after mixing continue to flow down a tube with a constant flow rate. Each particular distance down the tube then corresponds to a different time after mixing. Because the continuous-flow method requires a large amount of material, a more popular technique is that of stopped-flow. where the complete reaction is followed as a function of time in an observation chamber following the stopping of the solution flow. These techniques are limited by the time required for mixing, which is usually on the order of a millisecond. For the investigation of faster reactions another method must be used. Perturbation techniques involve the perturbation of a pre-existing equilibrium or steady- state. The perturbation could be caused by a sudden change in temperature (temperature- jump), pressure (pressure-jump), electric field (electric field jump), a laser flash (flash photolysis) or concentration (concentration-jump). After the perturbation there is a change in the position of equilibrium. However, the system requires time to adjust (i.e. to relax) to the new equilibrium position. Therefore, in perturbation techniques one observes the time course of the relaxation into the new equilibrium position. From the dependence of the time course of the relaxation on concentration, one can derive kinetic information about the rate constants and mechanisms of fast reactions. Perturbation kinetic methods can allow the investigation of reaction systems down into the microsecond range and sometimes beyond. Because of the much wider timescale that can be investigated, relaxation kinetic or pre- steady-state kinetic studies can yield directly detailed kinetic information on all of the kinetic steps of a complicated reaction mechanism. In contrast, steady-state kinetic studies only allow the overall rate of formation of the final product to be measured. 2. Relaxation Kinetic Theory For the analysis of relaxation kinetic data a more specialized mathematical treatment is generally used. For the development of the theory for the analysis of temperature-jump relaxation experiments as well as the technical development of temperature-jump apparatus capable of resolving the kinetics of reactions on the microsecond timescale Manfred Eigen (Max-Planck-Institute for Biophysical Chemistry, Gttingen) received the Nobel Prize in Chemistry in 1967. Let us consider first the application of the theory to a very simple reaction: A+B2 Consider the perturbation of the equilibrium at t=0 by a sudden change in temperature. This causes an instantaneous change in the values of ki and k., to new values. For the rest of the mathematical treatment we will consider ky and k., to represent the values at the new temperature following the jump. The differential rate equation describing the reaction can be written as: -kcjfB-k_ifc Alternatively the differential rate equation can be written as: d(Coqa + Ac) dt dt = k(C4 + ACXCqB + Acg)-k_1(Copc +Acc) (2) where the terms ACA. Aca and Ace represent the deviations in the concentrations from their final equilibrium values. However, based on the law of conservation of mass, the deviations on each side of the reaction must be equal, i.e. ACA = ACB = -Acc (3) Moreover, based on the equilibrium condition that the rates of the forward and backward reactions are equal, deqa/dt=0 and kicag.aCoa-kiCac=0. Taking these two facts and eq. (3) into consideration, (2) then reduces to: dac -= k (Copt + C)AC + k_j Ac+k (Ac) dt (1) (4) This is a nonlinear differential equation. However, for small perturbations, Acx < In A Ac = ~-[kr (Capt + Coqts) + k-1 ]t (6) Now we define the relaxation time, t, which is the time taken for ACA to decay to 1/e of its original value, i.e. at t=t, ACA = Aca/e. Concentration, [A] T Exponential relaxation T Time, t Substituting this condition into the integrated rate equation (6) yields that for this particular reaction mechanism t is related to the rate constants and the equilibrium concentrations of A and B by: == k (Cegt + Coq) + k_1 How should experimental data of 1/t and the concentrations of A and B be plotted to determine k, and k.? N.B. The important point is that in relaxation kinetic experiments the perturbation is kept small so that the observed kinetics are always first order. This simplifies greatly the mathematical analysis. In the case of a multistep mechanism, each individual reaction step should be associated with an exponential relaxation. In the case of first order reactions its not important how big the perturbation is, because they already follow first order kinetics. 3. Analysis of a Two-Step Mechanism The binding of adenosine diphosphate (ADP) to the enzyme creatine phosphotransferase has been investgated by the temperature jump method. A relaxation time in the range 60- 150 sec was observed. The data of the total ADP concentration and the reciprocal relaxation times measured were: CADP/MM 0.05 0.10 0.30 0.50 0.75 1.0 2.0 3.0 5.0 DAEADP dt ADP was far in excess of the enzyme concentration for all of the measurements. The relaxation effect was assumed to arise from a conformational change in the enzyme- ADP complex 1/t/s- 3.7 x 10 5.9 x 10 8.3 x 10 1.0 x 10 E+ADP (EADP) (EADP) which follows the rapid equilibrium of enzyme-ADP complex formation. The aim of this exercise is to evaluate from the data the values of k, k2 and K. Write down the differential rate equation expression for the change in the deviation of the concentration of (EADP) from its final equilibrium value, i.e. dAEADP;/dt = ? =kAEADP-k_AEADP 1.13 x 10 1.31 x 10 1.42 x 10 1.58 x 10 1.62 x 10 k2 (8) Write down the law of conservation of mass for the enzyme species, i.e. an expression showing that the sum of the changes of all of the enzyme species must be zero (the enzyme isn't disappearing). (9) The first step is considered to be in equilibrium on the time scale of the conformational change. Thus, write down the expression for the equilibrium constant K, rearrange to get [E] alone on the left hand side and differentiate with respect to EADP, to arrive at an expression relating AE to A(EADP). Because ADP was in excess, you can assume that [ADP] is a constant. (10) Now combine equations (8)-(10) to arrive at a linear first order differential equation in AEADP, and finally an expression of the reciprocal relaxation time. (11)