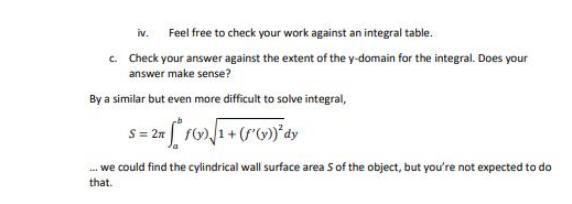
1. Consider a helical coil spring where a center position on its helix can be described by x = r coss, y =r sin s, and z=s, where x, y, and z are the Cartesian coordinates, and the independent variables (in radians) is used to locate the unique position on the spring's helix. The arc length formula becomes: --[0-0-0- + If the radius r = 1 cm, the pitch p = 0.75 cm, and the spring has exactly 12 coils, then find the uncoiled length of the material of the spring. How much extra material length is needed for the spring compared to the material needed for 12 rings of radius r? (Assume that the material length does not stretch or ca press upon forming the material into a spring or cutting it and forming it into rings.) 2. Now let's return to the problem posed in Discussion 1, but to compute the object's arc length across the curved surface, in any plane that also includes the symmetry axis. For minimum difficulty, we'll use the x, y plane. We'll have to use the formula: L = [1 + (F6))*dy a. Beginning with the same expression from Discussion 1, y = 2+22(3-x), either convert it to a function of x or use your result from Discussion 1. The integration domain should also be the same as in Discussion 1. Write the arc length integral over the y domain. b. Solve this integral, rounding your result to the nearest 0.01. Hints: i. ii. iii. For a term of the form a + w, where w is a dependent variable and a is a constant, make the trigonometric substitution w = a tan 8. You'll need to use integration by parts once the substitution above is made and the integral is simplified. Be sure to move the right-hand side of the equation term that is identical to the initial integral resulting from simplification of hint i, such that this integral is Feel free to check your work against an integral table. c. Check your answer against the extent of the y-domain for the integral. Does your answer make sense? iv. By a similar but even more difficult to solve integral, S = 2 *FG)1 + (())*dy ... we could find the cylindrical wall surface area 5 of the object, but you're not expected to do that.