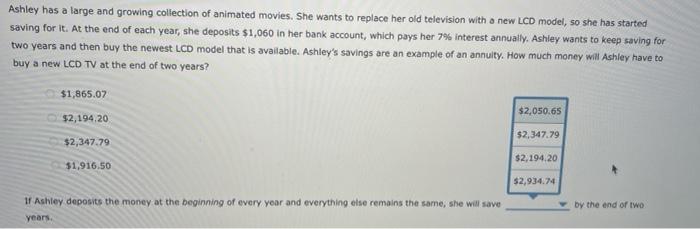
Ashley has a large and growing collection of animated movies. She wants to replace her old television with a new LCD model, so she has started saving for it. At the end of each year, she deposits $1,060 in her bank account, which pays her 7% interest annually. Ashley wants to keep saving for two years and then buy the newest LCD model that is available. Ashley's savings are an example of an annuity. How much money will Ashley have to buy a new LCD TV at the end of two years? $1,865.07 $2,050.65 $2,194.20 $2,347.79 $2,347.79 $2,194.20 31,916.50 $2,934.74 If Ashley deposits the money at the beginning of every year and everything else remains the same, she will save years , by the end of two 4. Present value of annuities and annuity payments The present value of an annuity is the sum of the discounted value of all future cash flows. You have the opportunity to invest in several annuities. Which of the following 10-year annuities has the greatest present value (PV)? Assume that all annuities earn the same positive interest rate. An annuity that pays $500 at the end of every six months An annuity that pays $1,000 at the beginning of each year An annuity that pays $1.000 at the end of each year An annuity that pays $500 at the beginning of every six months An ordinary annuity selling at $8,860.53 today promises to make equal payments at the end of each year for the next six years (N). If the annuity's appropriate interest rate (1) remains at 8.00% during this time, the annual annuity payment (PMT) will be You just won the lottery. CongratulationsThe Jackpot is $10,000,000, pald in six equal annual payments. The first payment on the lottery jackpot will be made today. In present value terms, you really won assuming annual interest rate of 8.00% Perpetuities are also called annuities with an extended or unlimited life. Based on your understanding of perpetuities, answer the following questions. Which of the following are characteristics of a perpetuity? Check all that apply. The value of a perpetuity cannot be determined. A perpetuity is a stream of regularly timed, equal cash flows that continues forever. The current value of a perpetuity is based more on the discounted value of its nearer (in time) cash flows and less by the discounted value of its more distant (in the future) cash flows. The value of a perpetuity is equal to the sum of the present value of its expected future cash flows. A local bank's advertising reads: "Give us $45,000 today, and we'll pay you $800 every year forever." if you plan to live forever, what annual interest rate will you earn on your deposit? 2.49% 2.85% 1.42% 1.78% Oops! When you went in to make your deposit, the bank representative said the amount of required deposit reported in the advertisement was Incorrect and should have read $67,500. This revision, which will the interest rate earned on your deposited funds, will adjust your earned interest rate to After Shipra got a job, the first thing she bought was a new car. She took out an amortized loan for $20,000 with no ($0) down payment. She agreed to pay off the loan by making annual payments for the next four years at the end of each year. Her bank is charging her an interest rate of 6% per year. Yesterday, she called to ask that you help her compute the annual payments necessary to repay her loan. Calculate the annual payment and complete the following loan amortization table: Year Payment Interest Paid Beginning Amount $20,000.00 Principal Pald Ending Balance 1 2 3 30.00