Assignment Questions:-
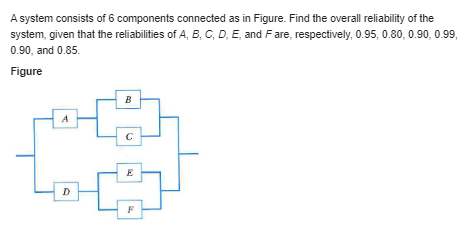
To investigate the average time to failure of a certain weld subjected to continuous vibration, 7 welded pieces were subjected to specified frequencies and amplitudes of vibration and their times to failure were 211, 350, 384, 510, 539, 620, and 715 thousand cycles. (a) Assuming the exponential model, construct a 95% confidence interval for the mean life (in thousands of cycles) of such a weld under the given vibration conditions. (b) Assuming the exponential model, test the null hypothesis that the mean life of the weld under the given vibration conditions is 500,000 cycles against the two-sided alternative / # 500,000. Use the level of significance 0.10.(Stress-strength models for reliability) An alternative model used in reliability treats the environmental stress as a random variable X, with probability density ((x), and the strength of the component to withstand this stress as an independent random variable Y having probability density g()). Then, the reliability is defined as R = P(Y > X] = fx) gly)dxdy -00 = where F(x) is the distribution function of X. Evaluate this reliability when (a) X has an exponential distribution with o = 0.01 and Y has an exponential distribution with failure rate 0.005; (b) X has an exponential distribution with o = 0.005 and Y has an exponential distribution with failure rate 0.005; (c) In X has a normal distribution with / = 60 and o = 5 and In Y has a normal distribution with p = 80 and o = 5.Using the fact that 2 Tr /p is a value of a random variable having the chi square distribution with 2 r degrees of freedom, derive the confidence interval for / given on page 494.A system consists of 6 components connected as in Figure. Find the overall reliability of the system, given that the reliabilities of A, B, C, D, E, and Fare, respectively, 0.95, 0.80, 0.90, 0.99, 0.90, and 0.85. Figure B A C E DSuppose that the flight of an aircraft is regarded as a system having the three main components A (aircraft), B (pilot), and C (airport). Suppose, furthermore, that component B can be regarded as a parallel subsystem consisting of 1(captain), 82 (first officer), and 83 (flight engineer); and C is a parallel subsystem consisting of C1 (scheduled airport) and C2 (alternate airport). Under given flight conditions, the reliabilities of components A, 81, 82, 83, C1, and C2 (defined as the probabilities that they can contribute to the successful completion of the scheduled flight) are, respectively, 0.9999, 0.9995, 0.999, 0.20, 0.95, and 0.85. (a) What is the reliability of the system? (b) What is the effect on system reliability of having a flight engineer who is also a trained pilot, so that the reliability of 83 is increased from 0.20 to 0.99? (c) If the flight crew did not have a first officer, what then would be the effect of increasing the reliability of 83 from 0.20 to 0.99? (d) What is the effect of adding a second alternate landing point, C3, with reliability 0.80?\fIn some reliability problems we are concerned only with initial failures, treating a component as if (for all practical purposes) it never fails, once it has survived past a certain time t = o. In a problem like this, it may be reasonable to use the failure rate for 0